Task 1: Explain the difference between linear and quadratic equations. Provide examples of each type of equation and describe their general forms.
Let's begin with linear equations, examples, and their form before looking at quadratic equations, examples, and their forms.
Liner Equations
It is an equation that represents straight lines when it is graphed, and the general form is ax + b = 0 where a = 0. In this equation, the variable of x is 1 and it is the highest power of variable. Also, in addition to this, linear equations have a constant rate of change and describe a linear relationship.
Example of Linear Equation:
| Linear equation | 3x - 5 = 0 |
|---|---|
| General form | az + b = 0 |
| Solution | x = 5/3 |
Quadratic Equations
It is an equation that represents parabolas curved when it is graphed, and the general form is ax2 + bx + C = 0 where a = 0. In this equation, the variable of x is 2 and it is the highest power of the variable. Also, in addition to what we have said, quadratic equation scenarios with decelerating or accelerating rates of change.
Example of Quadratic Equations
| Quadratic equation | 2x - 4x + 3 = 0 |
|---|---|
| General form | ax2 + bx + c = 0 |
| Solution | x = 1 and x = 3 will be calculated as (x-1)(x-3) = 0) |
Graphical Representation
- Linear equation y = 2x + 1
- Quadratic equation if a > 0 upward, if a < 0 downward.
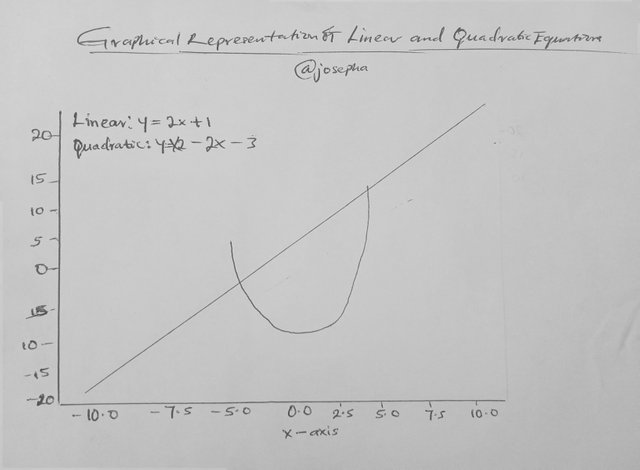
From the graphical representation above, you can see that the linear equation produces a straight line. On the other hand, the quadratic equation produces a parabolic that opens downwards or upward.
Task 2: Describe two different types of inequalities(Which are not explained in the course). Provide examples of each type of inequality and explain how to solve them.
The two different types of inequality that are not explained in the professor course that we will be describing are: absolute value and rational inequalities.
Absolute Value Inequalities
This is an inequalities that require special techniques to solve since it involves absolute value.
Example:
Solve |x - 3| < 5
Solution
We will have to rewrite the inequality without repeating the absolute value: -5 < x - 3 < 5
At this point, we will have to split into two separate inequalities:
x - 3 > -5
x - 3 < 5We can then solve the both inequalities:
x > - 2
x < 8Let's then combine the solutions:
- 2 < x < 8
Rational Inequalities
This is an inequality where the denominator or numerator is an algebraic expression.
Example
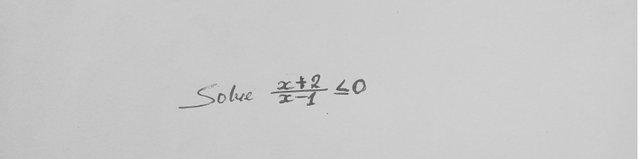
Based on the given example above, we are going to solve the problem based on the steps in the provided solutions.
Solution
I will need to identify the critical points by setting the denominator and numerator to zero.
Denominator: x - 1 = 0 → x = 1
Numerator: x + 2 = 0 → x =-2
At this point, we will have to divide the number line into intervals based on the points: (- ∞, - 2), (-2, 1), (1, ∞)
Now we will have to test the values in each interval to determine where the inequality is true as shown below 👇.
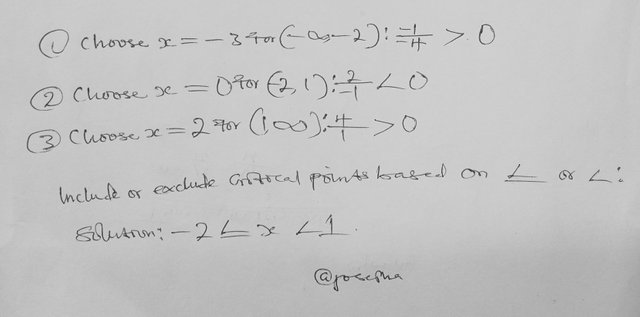
From the above, we can see what the rational inequality is.
Task 3 Solve the following linear equation: 2x + 5 = 11. Show step-by-step solutions and share practical examples of how this equation can be applied in real-life scenarios.

From the above, our final answer is x = 3
Practical example
Scenario:
Imagine I went to a bookshop to buy a book, and I am buying books, where each costs $2. Also, I have to pay a fixed shipping fee of $5. As a retailer, I want to know how many books I can buy if my total budget is $11.
Based on the given example above, we will then express the information as;
- Let x = to the number of books
- Total cost equation = 2x + 5 = 11
- By solving x = 3 it means that I can buy 3 books within my budget.
Task 4: Scenario: Tom's Bakery
Tom's Bakery is the most famous bakery of two and it's famous for its yummiest pastries and bread. Tom the owner wants to make sure that he has sufficient ingredients to meet the needs of his famous chocolate cake and chocolate cake recipe 2 cups of flour, 1 cup of sugar, and 1/2 cup of cocoa powder for each cake.
Based on the given scenario provided by the professor let's solve gh equation and inequality.
Equation:
The equation states that if Tom has the following:
- 10 cups of flour
- 8 cups of sugar
- 4 cups of cocoa powder and each cake requires the following:
- 2 cups of flour
- 1 cup of sugar
- 1/2 cup of cocoa powder
Let's now calculate the number of cakes x that can be made by finding the limiting ingredient.
Flour Constraint
- 2x ≤ 10
- x ≤ 10 / 2
- x = 5
Sugar Constraints
x ≤ 8
Now since each cake requires 1 cup of sugar it will be expressed as 8/1 = 8
Cocoa Powder Constraints:
- 0.5x ≤ 4
- x ≤ 4/0.5
- x ≤ 8
Based on the above, Tom can make a maximum number of 5 cakes.
Inequality
According to the information and what we have calculated so far, Tom has 8 cups of sugar and he wants to make at least 6 cakes Since each cake needs 1 cup of sugar, we will state it as:
- x ≥ 6 and x ≤ 8
By combining the above inequality we will then have
- 6 ≤ x ≤ 8
Our final answers are:
- Equation: 5 cakes
- Inequality: if it is at least 6 cakes that Tom wants to make, he will need between 6 cups of sugar and 8 cups of sugar.
Scenario: Number of gallons Ashley needs.
Suppose there's a person named Ashley who is planning a road trip from City A to City B. If I talk about distance between two cities then it's around 240 miles. Ashley's car fuel tank can hold 12 gallons Suppose that his car gets 20 miles for each gallon then how many gallons of gas may Ashley purchase if he already has 2 gallons in his tank and he wants to have a minimum of 1 gallon left over when he arrives at City B?
Based on the question the inequality step can be solved as follows
Problem Setup
| The distance between cities A and B | 240 miles |
|---|---|
| Car's mileage | 20 miles per gallons |
| Gas tank: Ashley | 2 gallons |
| Minimum leftover gas at City B | 1 gallon |
Inequality Setup
The total gallons needed for the trip will be calculated as:
Gas needed for the trip: Total Miles / Miles per gallon = 240 / 20 12 gallons
Total gas Ashley has after purchasing gallon x:
Gas in tank already + Gas Purchased - Gas Used ≥ Leftover gas
At this point, we will have to substitute the value
x + 2 ≥ 12 - 1
Let's simplify
x + 2 ≥ 11
Subtract 2 from both sides: x ≥ 9
Based on what we have calculated it means that Ashley needs to purchase a minimum of 9 gallons of gas for the trip to be completed and have at least a remaining 1-gallon tank.
I am inviting; @dove11, @simonmwigwe, and @ruthjoe
Cc:-
@khursheedanwar
Boss you have written well and I wish that sc01 vote on your post
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Thank you.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit