Welcome Here Friends
 |
|---|
Task 1
• Explain the difference between polynomial and rational expressions. Provide examples of each type of system of equation and describe their general forms.
Polynomial Expressions
This is known as a mathematical expression consisting of coefficients, variables, and nonnegative integer exponents that are combined using multiplication, subtraction, and addition. Also, it is an expression that does not contain variables in the under radicals or denominators.
General Form:
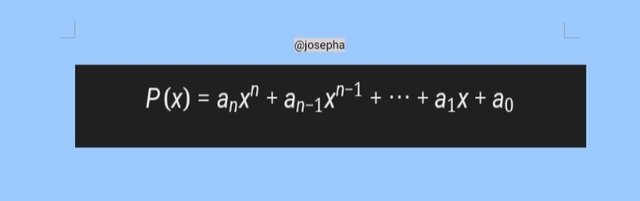
Looking at the above general form it is expressed as seen below.
Example:
P(x) = 3x^3 + 2x^2 - 5x + 7
Rational Expressions:
This is known as a ratio of two polynomial expressions. It is an expression that is expressed in the form of a fraction, where both the denominator and the numerator are polynomials.
General Form:

The general form is expressed as seen above.
Example:
R(x)= 2x^2 + 3x - 4 / x−1
System of Equation Examples
Polynomial System of Equations:
In polynomial system of equations involves the given example below.
Rational System of Equations
Example
2x^2 + y^2 = 5
x + y = 3
In rational the system of equations involves rational expressions as shown in the given example.
Example:
x/y + y/x = 3
x^2 - 1/y + 2 = 2
In summary, both can be used in the system of equations to model many real-world problems, but as for rational expressions, adds add complexity due to denominator ≠ 0 which is restricted. In addition to this polynomials are much simpler expressions, while rational expressions can involve division by a polynomial.
Task 2
• Explain steps used in simplifying a rational expression. Write some common factors required to be canceled out.
Let's take a look at the steps for simplifying a rational expression:
- Factoring the numerator and denominator
At this point, we will have to break down the both denominator and numerator into their simplest factors. Example using common factor, or formulas like a^2 - b^2 = (a - b)(a + b))
Example:

From the above example, we will have to identify the common factors by looking for terms that are present in both the denominator and numerator.
Example:
(x - 2)(x + 2 ) / (x + 1)(x + 2) The common factor to this is (x + 2)
- Cancel the Common Factors:
We will have to divide the denominator and numerator by their greatest common factor.
Example
(x - 2)(x + 2) / (x + 1)(x + 2) = (x - 2)(x + 1)
- Simplify the remaining expression
We will have to rewrite the expression without us canceling the terms.
Example:
Simplified expression: x - 2 / x + 1
- State the restrictions:
Let's determine the value that makes the denominator zero in the original expression since division by zero is undefined.
Example:
x^2 - 4 / x^2 + 3x + 2, restrictions are x + 2 → 0 or, x + 1 = 0, i.e., = -2, -1.
Common Factors to Be Canceled
- Binomial Factors:
x + a or x - b
Example:
(x + 3)(x - 2) / (x + 3)(x + 5)→ x - 2 / x + 5
- Common Monomials:
ax^n or constants like 2, 3.
Example:
4x^2 / 2x →2x / 1 = 2x
- Special Forms:
The difference of square : a^2 - b^2 = (a - b)(a + b)
Example: x^2 - 9 / x^2 - x - 6 → (x - 3)(x + 3) / (x - 3) (x + 2) = x + 3 / x + 2
- Common Factors In Polynomial:
Factored that can be a further factor.
Example: x^2 + 2x / x^3 + x^2 → x(x + 2) / x^2(x + 1) →x + 2 / x(x + 1)
If we can follow the above steps, we can systematically simplify any rational expression if given.
Task 3

Task 4
Scenario number 1
- Our first step will be to write the basic cost expression
$5 is the fixed cost, and the cost for each packet is $2. For x packets, the total basic cost before any discounts can be written as:
Where:
5 stands for fixed cost
2x stand for variable cost for x packets
Apply the 10% discount
Here we are going to reduce 10% the total cost which will then become:
Simplify the expression:
C = (5 + 2x) × 0.9
Distribute 0.9 across 5 + 2x:
C = 0.9 × 5 + 0.9 × 2x
C = 4.5 + 1.8x
- Calculate the total cost for 5 packets of bread
Let's substitute x = 5 into the discounted cost expression:
C = 4.5 + 1.8 × 5
Calculate 18 × 5
1.8 × 5 = 9
Add 4.5 + 9:
C = 13.5
Final Outcome
The polynomial expression that is representing the cost cost is C = 4.5 + 1.8x. If Ali buys 5 packets of bread the total cost will be C = $13.5 dollars
Scenario number 2
- Write the expression for the total harvest down
- Tons of wheat = x
- Tons of barley= 3x
Total harvest is = x + 3x = 4x
- Write the rational expression for the ratio of wheat to the total harvest
Ratio= Wheat / Total Harvest = x / 4x
- Simplify the expression
Cancel x as long as x = 0
Ratio = 1 / 4
This means the simplified expression for the ratio of wheat to total harvest is then = 1/4
- Calculate the ratio when the farmer harvests 4 tons of wheat
Let's substitute x = 4 into the expression for wheat and the total harvest.
- Wheat = x = 4 tons
- Total harvest= 4x = 4 × 4 = 16 ton
The ratio is: 4/16 = 1/4
Final Outcome
1/4 is the simplified rational expression for the ratio of wheat to total harvest. However, if the farmer harvests 4 tons of wheat, the ratio remains 1/4.
I am inviting; @dove11, @lhorgic, and @ruthjoe
Cc:-
@khursheedanwar