In Book 1 of Euclid’s Elements, Definition 13 reads (Fitzpatrick 6):
| Greek | English |
|---|---|
| ιγʹ. ̔́Ορος ἐστίν, ὅ τινός ἐστι πέρας. | 13. A boundary is that which is the extremity of something. |
Other translators have translated τινός [tinos] as of anything rather than of something (Heath 182). Both translations are valid, but do they mean the same thing?
David E Joyce calls Definition 13 a “rather nebulous” definition, as it involves an undefined term, πέρας [peras] or extremity. Euclid assumes that we already know what an extremity is. In this definition, then, is he simply telling us that the technical term for an extremity in Euclidean geometry is boundary? Or is he introducing this term to describe a particular type of extremity? Is boundary a species and extremity a genus?
As Heath points out, both words ὅρος [horos] and πέρας are used synonymously by Aristotle (Heath 182):
For this reason the bones on the one hand only grow up to a certain point (for there is a limit [πέρας] of size in all animals, and therefore also of the growth of the bones; if these had been always able to grow, all animals that have bone or its analogue would grow as long as they lived, for these set the limit [ὅρος] of size to animals. (Barnes 2519 : Generation of Animals 2:6 : Aristotle 745a 6, 9)

Euclid has already defined the extremities—πέρατα [perata], which is the plural of πέρας—of a line as points (Definition 3) and the extremities of a surface as lines (Definition 6). Again, in those definitions he simply assumed that his readers would know what he meant by “extremities”. Does this mean that a “boundary” is a special case of extremity? In his commentary on Euclid, Proclus suggests that this is the case:
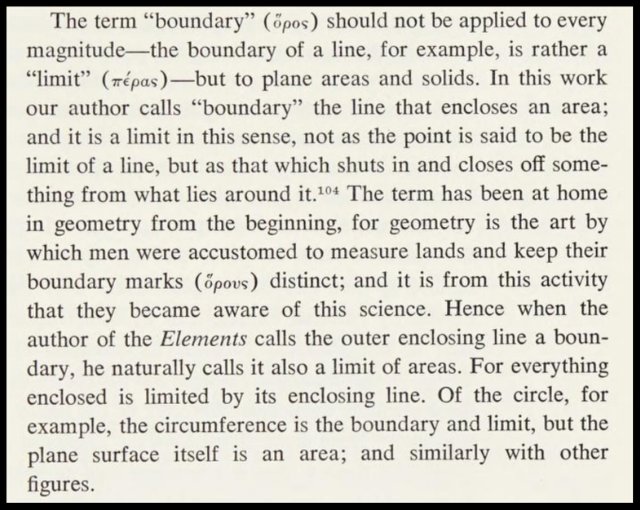
Glenn Morrow, the translator of this passage, comments:
104 136.8 Proclus wishes to emphasize, first, that ὅρος and πέρας are not synonymous—the latter being the more general term, for which reason it is used as genus in this definition—and, secondly, that ὅρος had from the beginning a special application to areas, as it has in the definition that immediately follows. Aristotle, however, uses the two terms as synonymous... (Morrow 109)
I presume this is why Morrow translates τινός as of something rather than of anything. In his French translation of the Elements, François Peyrard also translates τινός as something (quelque chose) rather than anything (n’importe quoi) (Peyrard 2).
I think it is safe to conclude that for Euclid a boundary is a special kind of extremity—just as Proclus surmises.
But it is not terribly important what position we adopt: Euclid only defines the term so that he can use it in Definition 14: A figure is that which is contained by some boundary or boundaries (Fitzpatrick 6). The term is never used again anywhere in the Elements.
And that’s a good place to stop.
References
- Jonathan Barnes (editor), The Complete Works of Aristotle, Volumes 1 & 2, Princeton University Press, Princeton, NJ (1984)
- Richard Fitzpatrick (translator), Euclid’s Elements of Geometry, University of Texas at Austin, Austin, TX (2008)
- Thomas Little Heath (translator & editor), The Thirteen Books of Euclid’s Elements, Second Edition, Dover Publications, New York (1956)
- Johan Ludvig Heiberg, Heinrich Menge, Euclidis Elementa edidit et Latine interpretatus est I. L. Heiberg, Volumes 1-5, B G Teubner Verlag, Leipzig (1883-1888)
- Henry George Liddell, Robert Scott, A Greek-English Lexicon, Eighth Edition, American Book Company, New York (1901)
- Glenn R Morrow (translator), Proclus: A Commentary on the First Book of Euclid’s Elements, Princeton University Press, Princeton, NJ (1970)
- François Peyrard, Les Œuvres d’Euclide, en Grec, en Latin et en Français, Volumes 1-3, Charles-Frobert Patris, Paris (1814, 1816, 1818)
Online Resources
