In Book 1 of Euclid’s Elements, the fourth of the Five Common Notions reads (Fitzpatrick 7):
| Greek | English |
|---|---|
| δʹ. Καὶ τὰ ἐφαρμόζοντα ἐπ ̓ ἀλλήλα ἴσα ἀλλήλοις ἐστίν. | 4. And things coinciding with one another are equal to one another. |
In some modern editions of the Elements this is listed as the eighth Common Notion (Billingsley 7 : August 4 : Peyrard 6). In Heiberg’s definitive text of 1883, it appears as the fourth of the five Common Notions, but Heiberg assigns it the number VII. In Proclus’s Commentary on the First Book of Euclid’s Elements, it is listed as the fifth Common Notion, though Proclus refers to them as axioms, or ἀξιώματα [axiomata] (Morrow 152, fn 23).
This Common Notion defines what has come to be known as Euclid’s Method of Superposition:
C.N.4 requires interpretation. On the face of it, it seems to say that if two things are identical (that is, they are the same one), then they are equal, in other words, anything equals itself. But the way it traditionally is interpreted is as a justification of a principle of superposition, which is used, for instance, in proposition I.4. Using this principle, if one thing can be moved to coincide with another, then they are equal. (Joyce)
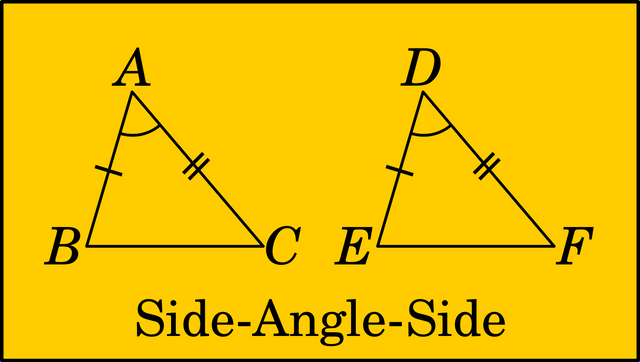
Euclid’s Method of Superposition has been criticized as one of the weaker aspects of his geometry:
The method of proof used in this proposition [Proposition 1:4] is sometimes called “superposition.” It apparently is not a method that Euclid prefers since he so rarely uses it, only here in I.4 and in I.8 and III.24, but not in many other propositions in which he could have used it.
It is not clear what is meant by “superposing a triangle on a triangle” means. It has been variously interpreted as actually moving one triangle to cover the other or as simply associating parts of one triangle with parts of the other. For the two triangles illustrated in the figure, you can actually slide one over the other in a continuous motion within the plane. Note, however, that if one triangle is the mirror image of the other, then any continuous motion would require moving one triangle outside of the plane. But the triangles don’t have to be same plane to begin with, and they often are not in the same plane when this proposition is invoked in the books on solid geometry.
Whatever the intended meaning of superposition may be, there are no postulates to allow any conclusions based on superposition. One possibility is to add postulates based on a group of transformations of space, or if restricted to plane geometry, on a group of transformations of the plane. Charles Dodgson (a.k.a. Lewis Carroll) would have said that using group theory is not appropriate to an elementary exposition of Euclidean geometry. Heath has described a more elementary conservative basis in his commentary on this proposition.
Yet another alternative is to simply take this proposition as a postulate, or part of it as a postulate. For instance, Hilbert in his Foundations of Geometry takes as given that under the hypotheses of this proposition that the remaining angles equal the remaining angles. Then, Hilbert proves that the base equals the base. (Joyce)
In his discussion of Proposition 1:8, Joyce refers to the hazy method of superposition.

As we have seen, Euclid’s Common Notions are so called because they are not peculiar to geometry, but common to all the sciences. But this important distinction between the Common Notions and the Postulates has been questioned in the case of this particular Common Notion. The French mathematician Paul Tannery saw this more as a definition of geometric equality, or congruence, than as an axiom:
As for Common Notions 7 and 8 [= Common Notions 4 and 5 in modern texts], they also do not seem to me to belong to Euclid, despite the authority of Proclus. Number 7 has an indisputably geometric character which should have caused it to be excluded from common notions; on the other hand, it is difficult to see why it is not accompanied by its converse, at least for straight lines, a converse which Euclid must use in the first place. In fact, there is a definition of geometric equality here, a more or less sufficient definition, but there is no real axiom. (Tannery 167)
Heath’s Commentary
Thomas Little Heath devotes no less than seven pages to his commentary on Euclid’s Common Notion 4. He begins by noting an important distinction between two possible meanings of the Greek verb Euclid employs in this Common Notion:
The word ἐφαρμόζειν [epharmozein], as a geometrical term, has a different meaning according as it is used in the active or in the passive. In the passive, ἐφαρμόζεσθαι [epharmozesthai], it means “to be applied to” without any implication that the applied figure will exactly fit, or coincide with, the figure to which it is applied; on the other hand the active ἐφαρμόζειν is used intransitively and means “to fit exactly,” “to coincide with.” In Euclid and Archimedes ἐφαρμόζειν is constructed with ἐπί [epi] and the accusative, in Pappus with the dative. (Heath 224-225)

Heath believes that Euclid actually envisaged the movement of one geometric figure relative to another:
The phraseology of the propositions, e.g. 1.4 and 1.3, in which Euclid employs the method indicated, leaves no room for doubt that he regarded one figure as actually moved and placed upon the other. (Heath 225)
But not all mathematicians agree with this interpretation:
Mr Bertrand Russell observes (Encyclopaedia Britannica, Suppl. Vol. 4, 1902, Art. “Geometry, non-Euclidean”) that the apparent use of motion here is deceptive; what in geometry is called a motion is merely the transference of our attention from one figure to another. Actual superposition, which is nominally employed by Euclid, is not required; all that is required is the transference of our attention from the original figure to a new one defined by the position of some of its elements and by certain properties which it shares with the original figure. (Heath 227)
Today we would define superposition in terms of a composition of isometries. A given geometric figure, such as a triangle, would be subjected to various isometric transformations—translation, rotation, reflection in a line, etc—so that it comes to lie perfectly upon the other figure.
And that’s a good place to stop.
References
- Ernst Ferdinand August, Euclidis Elementa, Part 1, Theodor Trautwein, Berlin (1826)
- Henry Billingsley (translator), The Elements of Geometrie of the Most Auncient Philosopher Evclide of Megara, John Day, London (1582)
- Richard Fitzpatrick (translator), Euclid’s Elements of Geometry, University of Texas at Austin, Austin, TX (2008)
- Thomas Little Heath (translator & editor), The Thirteen Books of Euclid’s Elements, Second Edition, Dover Publications, New York (1956)
- Johan Ludvig Heiberg, Heinrich Menge, Euclidis Elementa edidit et Latine interpretatus est I. L. Heiberg, Volumes 1-5, B G Teubner Verlag, Leipzig (1883-1888)
- David Hilbert, E J Townsend (translator), Foundations of Geometry, Second Edition, The Open Court Publishing Company, Chicago (1910)
- Henry George Liddell, Robert Scott, A Greek-English Lexicon, Eighth Edition, American Book Company, New York (1901)
- Glenn Raymond Morrow (translator), Proclus: A Commentary on the First Book of Euclid’s Elements, Princeton University Press, Princeton, NJ (1970)
- François Peyrard, Les Œuvres d’Euclide, en Grec, en Latin et en Français, Volumes 1-3, Charles-Frobert Patris, Paris (1814, 1816, 1818)
- Paul Tannery, Sur l’authenticité des axiomes d’Euclide, Bulletin des Sciences Mathématiques et Astronomiques, Series 2, Volume 8, Number 1, Pages 162-175, Gauthier-Villars, Paris (1884)
- Thomas Taylor, The Philosophical and Mathematical Commentaries of Proclus on the First Book of Euclid’s Elements, Volume 1, Volume 2, London (1792)
Image Credits
- Paul Tannery: Anonymous Photograph, Public Domain
Online Resources
