In Book 1 of Euclid’s Elements, the fifth of the Five Common Notions reads (Fitzpatrick 7):
| Greek | English |
|---|---|
| εʹ. Καὶ τὸ ὅλον τοῦ μέρους μεῖζόν [ἐστιν]. | 5. And the whole [is] greater than the part. |
In some modern editions of the Elements this is listed as the ninth Common Notion (Billingsley 8 : August 4 : Peyrard 6). In Johan Ludvig Heiberg’s definitive text of 1883, it appears as the fifth of the five Common Notions that Heiberg considers genuine, but he assigns it the number VIII. In Proclus’s Commentary on the First Book of Euclid’s Elements, it is listed as the fourth of the Common Notions, though Proclus refers to these as axioms, or ἀξιώματα [axiomata] (Morrow 152, fn 23).
Heiberg notes that the verb ἐστιν [estin], is, is omitted by Proclus. François Peyrard has the form ἐστι, dropping the so-called movable nu. This letter is optional, but it is often used, as here, at the end of ἐστι (Smyth 34). Ernst Ferdinand August notes that one manuscript, V (Codex Vindobonensis Gr 103), has the present infinitive εἶναι [einai], to be (see also Peyrard 454). Heiberg only mentions P, Codex Vaticanus Gr 190, as having εἶναι.

Authenticity
The expression that the whole is greater than the part is one of the most recognizable quotations from Euclid’s Elements. Nevertheless, several scholars have questioned its authenticity. Proclus accepted it as a genuine Euclidean axiom, and the manuscript evidence generally supports this position. As we have seen, Heiberg also considered it one of the five authorial axioms. But Heron only recognized the first three Common Notions as authoritative (Morrow 154 : Tannery 166).
In the 19th century the French mathematician Paul Tannery argued that none of the Common Notions belonged to Euclid’s original text : they were all interpolated by later scholars:
As for the common notions 7 and 8, they also seem to me not to belong to Euclid either, despite the authority of Proclus ...
Finally statement 8 replaces a different expression of Euclid’s: Proposition VI: “The lesser will be equal to the greater, which is absurd”. It is an abstraction substituted for the intuitive interpretation of the geometric figure; an abstraction which, moreover, is reduced to a more or less insufficient definition of the whole and of the part, if one wants to break away from its geometric specialty and really make it a common notion. (Tannery 167)

The British mathematician Thomas Little Heath, who translated the Elements into English in the early 20th century, concurred:
Proclus includes this “axiom” on the same ground as the preceding one [ie it is required to prove some propositions]. I think however there is force in the objection which Tannery takes to it, namely that it replaces a different expression in Eucl. I.6, where it is stated that “the triangle DBC will be equal to the triangle ACB, the less to the greater: which is absurd.” The axiom appears to be an abstraction or generalisation substituted for an immediate inference from a geometrical figure, but it takes the form of a sort of definition of whole and part. The probabilities seem to be against its being genuine, notwithstanding Proclus’ approval of it. (Heath 232)
The Italian historian of philosophy and science Vincenzo De Risi has suggested that the fourth and fifth common notions were introduced by Theon of Alexandria, who published an edition of the Elements in the 4th century:
I show that, while CN1-CN3 are solidly embedded in the Euclidean text and often mentioned in the earlier Greek tradition, CN4 and CN5 are only tenuously connected with the text of the Elements and do not appear in other ancient sources before the fifth century CE. I conclude that the last two common notions are most probably spurious and I advance the conjecture that they were interpolated by Theon of Alexandria. (De Risi 302)
De Risi suggests that Euclid did not consider CN4 and CN5 as axiomatic precisely because they were inferences drawn from geometric diagrams:
The comparison of figures through CN4 and CN5 occurs by means of diagrammatic inferences, whereas CN1-CN3 are purely propositional principles. It is perfectly reasonable, then, that Euclid may have only allowed propositional axioms in the foundations of mathematics and regarded diagrammatic inferences as non-axiomatic. (De Risi 316)

Interpretation
David E Joyce has suggested that in Common Notion 5 Euclid is actually defining the mathematical concept of greater than:
C.N.5, the whole is greater than the part, could be interpreted as a definition of “greater than.” To say one magnitude B is a part of another A could be taken as saying that A is the sum of B and C for some third magnitude C, the remainder. Symbolically, A > B means that there is some C such that A = B + C. At any rate, Euclid frequently treats these two conditions as being equivalent. (Joyce)
This opinion is shared by some other mathematicians:
Euclid’s Common Notion 5, CN5 in short, reads:
CN5 “And the whole (ὄλον) [is] greater than the part (μέρους)”.
We argue that the following formula interprets this axiom:
(∀x, y)(x+y > x). Proposition II.14 [To construct a square equal in area to a given rectilinear figure] will play a key role in our argument. (Błaszczyk et al 73)
Vincenzo De Risi interprets this Common Notion in purely geometric terms:
Common notions CN4 and CN5 may be read as principles of a theory of content. Accordingly, CN4 would state that superposed figures are equal in content and CN5 that the whole is greater in content than the part. After the transformation of any polygonal figure into an equivalent square [Proposition 2:14] ... the resulting squares may be compared with one another by superposing them. Through superposition, we may order the figures in terms of content, by saying that a figure is greater in content than another if it contains the other. In order to make such a comparison, we need CN4 to say that the smaller figure, which is superposed to a part of the larger figure, is equal in content to such a part. We then need CN5 to conclude that the larger figure is greater in content than its part and therefore greater than the other figure. (De Risi 317)

Aristotle
This axiom is often confused with the popular saying:
The whole is greater than the sum of its parts.
This sentiment is usually attributed to Aristotle, who wrote something very close to it in his Topics (Book 6, Chapter 13):
In general, too, all the ways of showing that the whole is not the same as the sum of its parts are useful in meeting the type just described; for a man who defines in this way seems to assert that the parts are the same as the whole. The arguments are particularly appropriate in cases where the process of putting the parts together is obvious, as in a house and other things of that sort; for there, clearly, you may have the parts and yet not have the whole, so that parts and whole cannot be the same. (Barnes 252 : Aristotle 150a15 ff)
The translator Arthur Wallace Pickard-Cambridge—no doubt under the influence of the familiar expression—has translated Aristotle’s ἐπιχειρεῖν ὅτι οὐ ταὐτόν ἐστι τὰ μέρη καὶ τὸ ὅλον as showing that the whole is not the same as the sum of its parts. But the literal translation is: to attempt to prove that the parts and the whole are not the same. Aristotle never actually refers to the sum of its parts.
Aristotle also wrote something similar in his Metaphysics (Book 8, Chapter 6):
In the case of all things which have several parts and in which the whole is not, as it were, a mere heap, but the totality is something besides the parts, there is a cause of unity; for as regards material things contact is the cause in some cases, and in others viscidity or some other such quality. And a definition is a formula which is one not by being connected together, like the Iliad, but by dealing with one object. (Barnes 3547-3548 : Aristotle 1045a8 ff)
So Aristotle never wrote explicitly that the whole is greater than the sum of its parts. But he did come close.

Christopher Clavius
Thomas Heath concluded his brief analysis of this Common Notion with the following observation:
Clavius added the axiom that the whole is the equal to the sum of its parts. (Heath 232)
Christopher Clavius, a German Jesuit scholar of the late 16th century, is better known today as a geocentric astronomer, who resisted the Copernican Revolution and played a pivotal rôle in the introduction of the Gregorian Calendar. But he was also a mathematician and the author of a commentary on Euclid:
Euclidis elementorum libri XV : accessit XVI de solidorum regularium comparatione
The 15 Books of Euclid’s Elements : to which is added a 16th Book comparing the regular solids
The spurious 14th Book of the Elements was probably written by Hypsicles of Alexandria, a mathematician who flourished in the middle of the 2nd century BCE. Book 15 was also spurious and has been attributed, at least in part, to Isidore of Miletus, who flourished in the 6th century of the Common Era. Clavius took the liberty of adding a 16th book of his own to the Elements.
Here is what Clavius writes in his commentary on Common Notion 5, which is the ninth Common Notion in his edition of the Elements:
IX: Et totum sua parte maius est
Cum pars a toto ablata relinquat adhuc aliquid, ne totum ipsum auferatur; perspicuum est, omne totum sua esse parte maius.
9: And the whole is greater than its part.
When a part is taken away from the whole, let something still remain, lest the whole itself be taken away; It is clear that every whole is greater than its part.
Clavius added several other axioms to Euclid’s Common Notions. The one Heath had in mind is numbered XVIII:
XVIII: Omne totum æquale est omnibus suis partibus simul sumptis.
Quoniam omnes partes simul sumptæ constituunt totum, cuius sunt partes, manifesta veritas huius axiomatis.
18: Every whole is equal to all its parts taken together.
Since all the parts taken together constitute the whole of which they are the parts, the truth of this axiom is obvious.

Galileo on Common Notion 5
Clavius’s contemporary and rival Galileo Galilei also had something to say about Euclid’s Fifth Common Notion. He may have been the first mathematician, in fact, to cast doubt on the manifest truth of this statement.
Galileo did this in his final book, Discourses and Mathematical Demonstrations Relating to Two New Sciences, which was written when he was under house arrest, following his trial by the Roman Inquisition. It was published in 1638 in Leiden, a predominantly Protestant city in the Dutch Republic, where the writ of Rome meant little. Popularly known as Two New Sciences, this dialogue is concerned with the tensile strength of materials and the motion of objects. Galileo’s two new sciences are now known as material engineering and kinematics.
The passage in which he casts doubt on the validity of Euclid’s Fifth Common Notion is referred to as Galileo’s Paradox, and involves a discussion of the mathematical nature of infinity. Galileo’s mouthpieces—Simplicio, Salviati and Sagredo—have the following discussion:
Simplicio Here a difficulty presents itself which appears to me insoluble. Since it is clear that we may have one line greater than another, each containing an infinite number of points, we are forced to admit that, within one and the same class, we may have something greater than infinity, because the infinity of points in the long line is greater than the infinity of points in the short line. This assigning to an infinite quantity a value greater than infinity is quite beyond my comprehension.
Salviati This is one of the difficulties which arise when we attempt, with our finite minds, to discuss the infinite, assigning to it those properties which we give to the finite and limited; but this I think is wrong, for we cannot speak of infinite quantities as being the one greater or less than or equal to another. To prove this I have in mind an argument which, for the sake of clearness, I shall put in the form of questions to Simplicio who raised this difficulty.
I take it for granted that you know which of the numbers are squares and which are not.
Simplicio I am quite aware that a squared number is one which results from the multiplication of another number by itself; thus 4, 9, etc., are squared numbers which come from multiplying 2, 3, etc., by themselves.
Salviati Very well; and you also know that just as the products are called squares so the factors are called sides or roots; while on the other hand those numbers which do not consist of two equal factors are not squares. Therefore if I assert that all numbers, including both squares and non-squares, are more than the squares alone, I shall speak the truth, shall I not?

Simplicio Most certainly.
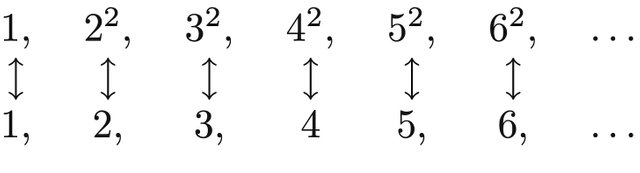
Salviati If I should ask further how many squares there are one might reply truly that there are as many as the corresponding number of roots, since every square has its own root and every root its own square, while no square has more than one root and no root more than one square.
Simplicio Precisely so.
Salviati But if I inquire how many roots there are, it cannot be denied that there are as many as the numbers because every number is the root of some square. This being granted, we must say that there are as many squares as there are numbers because they are just as numerous as their roots, and all the numbers are roots. Yet at the outset we said that there are many more numbers than squares, since the larger portion of them are not squares. Not only so, but the proportionate number of squares diminishes as we pass to larger numbers, Thus up to 100 we have 10 squares, that is, the squares constitute 1/10 part of all the numbers; up to 10,000, we find only 1/100 part to be squares; and up to a million only 1/1000 part; on the other hand in an infinite number, if one could conceive of such a thing, he would be forced to admit that there are as many squares as there are numbers taken all together.
Sagredo What then must one conclude under these circumstances?
Salviati So far as I see we can only infer that the totality of all numbers is infinite, that the number of squares is infinite, and that the number of their roots is infinite; neither is the number of squares less than the totality of all the numbers, nor the latter greater than the former; and finally the attributes “equal,” “greater,” and “less,” are not applicable to infinite, but only to finite, quantities. When therefore Simplicio introduces several lines of different lengths and asks me how it is possible that the longer ones do not contain more points than the shorter, I answer him that one line does not contain more or less or just as many points as another, but that each line contains an infinite number. (Galileo 31-33)
Galileo never explicitly mentions Euclid’s Fifth Common Notion in Two New Sciences, but it is implied in his Paradox, along with another important mathematical idea known as Hume’s Principle:
The concept of relative size with which Salviati and his author were equipped, taken in whole, cannot be applied consistently to infinite sets. For Galileo, these concepts involved at least two principles:
Euclid’s Principle (Common Notion 5): The whole is greater than the part (i.e., strictly greater than any proper part).
Hume’s Principle: Two collections are equal in numerosity if and only if their members can be put in one-to-one correspondence. [Footnote: Galileo did not spell out these principles, let alone call them by these names, but they are clearly implicit in his statement of the paradox.] (Parker 18)
Galileo did not pursue this subject any further. It was left to Bernard Bolzano, Georg Cantor and other mathematicians of the 19th and 20th centuries to take up the argument and develop a theory of infinite sets—the bane of modern mathematics.

And it is not only the infinitely large that has fallen foul of Euclid’s Fifth Common Notion. In the 18th century, when Gottfried Wilhelm Leibniz began to publish his theories on the infinitesimal calculus, the French mathematician Michel Rolle argued that the existence of infinitesimals—numbers closer to zero than any real number but not actually equal to zero—violated Euclid’s Common Notion 5 (Mancosu 230).
It is remarkable that so simple a statement of so obvious a truth has generated so much debate over the centuries.
And that’s a good place to stop.
References
- Ernst Ferdinand August, Euclidis Elementa, Part 1, Theodor Trautwein, Berlin (1826)
- Jonathan Barnes (editor), The Complete Works of Aristotle: The Revised Oxford Translation, Volume 1, Volume 2, Princeton University Press, Princeton, NJ (1984)
- Henry Billingsley (translator), The Elements of Geometrie of the Most Auncient Philosopher Evclide of Megara, John Day, London (1582)
- Piotr Błaszczyk et al, Decoding Book II of the Elements, Annales Universitatis Paedagogicae Cracoviensis: Studia ad Didacticam Biologiae Pertinentia, Volume 12, Pages 39-88, Pedagogical University of Cracow, Kraków (2020)
- Christopher Clavius, Commentary on Euclid, Vincentius Accoltus, Rome (1574)
- Richard Fitzpatrick (translator), Euclid’s Elements of Geometry, University of Texas at Austin, Austin, TX (2008)
- Galileo Galilei, Dialogues Concerning Two New Sciences, Translated from the Italian and Latin into English by Henry Crew & Alfonso de Salvio, The Macmillan Company, New York (1914)
- Thomas Little Heath (translator & editor), The Thirteen Books of Euclid’s Elements, Second Edition, Dover Publications, New York (1956)
- Johan Ludvig Heiberg, Heinrich Menge, Euclidis Elementa edidit et Latine interpretatus est I. L. Heiberg, Volumes 1-5, B G Teubner Verlag, Leipzig (1883-1888)
- Henry George Liddell, Robert Scott, A Greek-English Lexicon, Eighth Edition, American Book Company, New York (1901)
- Paolo Mancosu, The Metaphysics of the Calculus: A Foundational Debate in the Paris Academy of Sciences, 1700-1706, Historia Mathematica, Volume 16, Issue 3, Pages 224-248, Elsevier, Amsterdam (1989)
- Glenn Raymond Morrow (translator), Proclus: A Commentary on the First Book of Euclid’s Elements, Princeton University Press, Princeton, NJ (1970)
- Matthew W Parker, Philosophical Method and Galileo’s Paradox of Infinity, Bart Van Kerkhove (editor), New Perspectives on Mathematical Practices: Essays in Philosophy and History of Mathematics, World Scientific, Singapore (2009)
- François Peyrard, Les Œuvres d’Euclide, en Grec, en Latin et en Français, Volumes 1-3, Charles-Frobert Patris, Paris (1814, 1816, 1818)
- Vincenzo De Risi, Euclid’s Common Notions and the Theory of Equivalence, Foundations of Science, Volume 26, Issue 2, Pages 301-324, Springer Science+Business Media, Berlin (2021)
- Michel Rolle, Du Nouveau Systême de l’Infini, Histoire et Mémoires de Mathématique et de Physique de l’Académie Royale des Sciences, Pages 312-336, Paris (1703)
- Herbert Weir Smyth, A Greek Grammar for Colleges, American Book Company, New York (1920)
- Paul Tannery, Sur l’authenticité des axiomes d’Euclide, Bulletin des Sciences Mathématiques et Astronomiques, Series 2, Volume 8, Number 1, Pages 162-175, Gauthier-Villars, Paris (1884)
- Thomas Taylor, The Philosophical and Mathematical Commentaries of Proclus on the First Book of Euclid’s Elements, Volume 1, Volume 2, London (1792)
Image Credits
- Euclid: Common Notion 5: Jusepe di Ribera (artist), Getty Center, Los Angeles, Public Domain
- Paul Tannery: Anonymous Photograph, Public Domain
- Vincenzo De Risi: © Université Paris-Cité, Fair Use
- Aristotle: Plato (Leonardo da Vinci) & Aristotle (Giuliano da Sangallo?) in The School of Athens, Raphael (artist), Apostolic Palace, Vatican City, Public Domain
- Christopher Clavius: Francesco Vilamena (artist), Metropolitan Museum of Art, New York, Public Domain
- Galileo Galilei: Justus Sustermans (artist & engraver), Royal Museums Greenwich, London, Public Domain
- Michel Rolle, Modern Artist’s Impression of Michel Rolle, © Andreas Strick (artist), Fair Use
Online Resources
- Aristotle and The Whole Is Greater ...
- Euclid’s Elements by David E Joyce
- Life of Euclid
- Reading Euclid
