In Book 1 of Euclid’s Elements, Postulate 1 reads (Fitzpatrick 7):
| Greek | English |
|---|---|
| αʹ. ̓Ηιτήσθω ἀπὸ παντὸς σημείου ἐπὶ πᾶν σημεῖον εὐθεῖαν γραμμὴν ἀγαγεῖν. | 1. Let it have been postulated to draw a straight-line from any point to any point. |
In the last article in this series, we examined in some detail the meaning Euclid gave to the technical term αἴτημα [aitēma], postulate.
The verb ᾐτήσθω [ēitēsthō] is the third person singular, present perfect tense, imperative mood, middle voice (passive) of the verb αἰτέω [aitéō]: I postulate, I assume : I crave, I ask for. The translator Richard Fitzpatrick adds the following comment on Euclid’s use of this unusual form of the verb:
The Greek present perfect tense indicates a past action with present significance. Hence, the 3rd-person present perfect imperative Ηιτήσθω could be translated as “let it be postulated”, in the sense “let it stand as postulated”, but not “let the postulate be now brought forward”. The literal translation “let it have been postulated” sounds awkward in English, but more accurately captures the meaning of the Greek. (Fitzpatrick 7 fn)
| ᾐτήσθω | Let it have been postulated |
|---|---|
| Verb | αἰτέω |
| Tense | Present Perfect |
| Mood | Imperative |
| Voice | Middle (Passive) |
| Number | Singular |
| Person | Third |
I believe that Euclid uses this awkward form of the verb to reflect his Platonic philosophy. For Euclid, the fundamental truths and objects of geometry are like the perfect, eternal Forms or Ideas of Plato’s philosophy. These postulates were not invented by Euclid or one of his predecessors at a particular point in time. They have existed from time immemorial. If these eternal truths were ever postulated, it was God who postulated them when he created the world.
Note that Euclid does not bother to repeat this verb in the following four Postulates, but simply connects the latter to the First Postulate with the conjunction Καὶ [kai], and. The verb is understood to be the same.
In Thomas Heath’s translation, he actually separates the verb from the First Postulate, so that it serves as the antecedent of all Five Postulates:

In his 1814 edition of the Elements, François Peyrard spells this word ̓Ητήσθω [Ētēsthō], without the iota (ι [i]) following the initial eta (η [ē]). This spelling is also found in Ernst Ferdinand August’s 1826 edition. In Euclid’s day, the digraph ηι [ēi] represented a long diphthong, but during the Hellenistic period this diphthong became a pure vowel, η [ē]. To indicate the former diphthongal pronunciation Byzantine philologists invented the diacritic known as the iota subscript in the 12th century. This usually consisted of a small iota written beneath the preceding vowel, but not always:
The alternative practice, of writing the mute iota not under, but next to the preceding vowel, is known as iota adscript. In mixed-case environments, it is represented either as a slightly reduced iota (smaller than regular lowercase iota), or as a full-sized lowercase iota. In the latter case, it can be recognized as iota adscript by the fact that it never carries any diacritics (breathing marks, accents). (Wikipedia)
Discussion
It is a measure of the complexity of Euclidean geometry that even this brief postulate has been the subject of much discussion. Heath, for example, begins his commentary by pointing out how Euclid writes from every point to every point [ἀπὸ παντὸς σημείου ἐπὶ πᾶν σημεῖον] where we today would write from any point to any point:
From any point to any point. In general statements of this kind the Greeks did not say, as we do, “any point,” “any triangle” etc., but “every point,” “every triangle” and the like. Thus the words are here literally “from every point to every point.” Similarly the first words of Postulate 3 are “with every centre and distance,” and the enunciation, e.g., of 1.18 is “In every triangle the greater side subtends the greater angle.” (Heath 195)
The fundamental meaning of this postulate is not difficult to grasp:
This first postulate says that given any two points such as A and B, there is a line AB which has them as endpoints. (Joyce)

In the First Postulate, Euclid’s εὐθεῖα γραμμή [eutheia grammē], straight-line, refers to a finite line segment. The Second Postulate makes this clear. Elsewhere in the Elements, Euclid uses the term εὐθεῖα γραμμή to refer to both finite line-segments and infinitely extended lines—eg Definition 1:4: A straight-line is (any) one which lies evenly with points on itself.
The First Postulate asserts the existence of a line (ie line-segment) AB, but it does not say whether this line is unique. As it happens, this line is unique: given any two distinct points, there exists one and only one straight line-segment joining them:
Although it doesn’t explicitly say so, there is a unique line between the two points. Since Euclid uses this postulate as if it includes the uniqueness as part of it, he really ought to have stated the uniqueness explicitly. (Joyce)
Heath believes that this omission by Euclid led later commentators to add the so-called Sixth Postulate which appears in several old manuscripts of the Elements and which we discussed in the last article:
There is still something more that must be inferred from the Postulate combined with the definition of a straight line, namely (3) that the straight line joining two points is unique: in other words that, if two straight lines (“rectilineal segments,” as Veronese would call them) have the same extremities, they must coincide throughout their length. The omission of Euclid to state this in so many words, though he assumes it in 1.4, is no doubt answerable for the interpolation in the text of the equivalent assumption that two straight lines cannot enclose a space, which has constantly appeared in mss. and editions of Euclid, either among Axioms or Postulates. That Postulate 1 included it, by conscious implication, is even clear from Proclus’ words in his note on 1.4 (p. 139, 16) : “therefore two straight lines do not enclose a space, and it was with knowledge of this fact that the writer of the Elements said in the first of his Postulates, to draw a straight line from any point to any point, implying that it is one straight line which would always join the two points, not two.” (Heath 195 : Morrow 187)
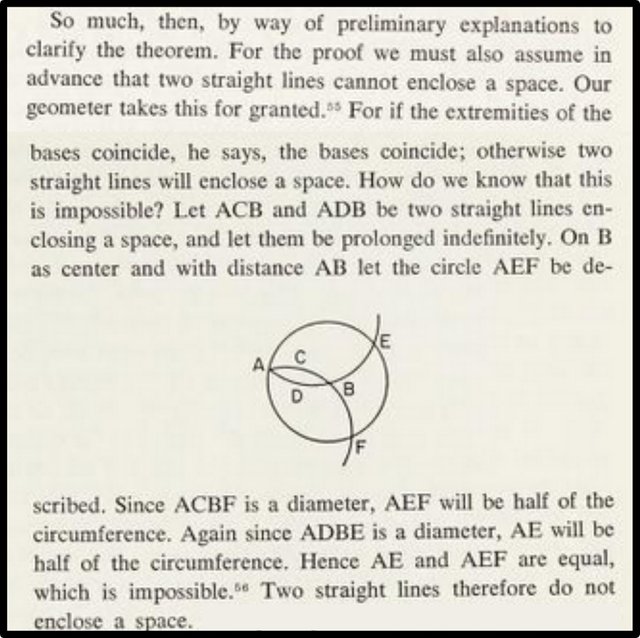
Proclus attempts to prove that two straight lines cannot enclose a space, but Heath refutes this proof:
It will be observed, however, that the straight lines produced are assumed to meet the circle given in two different points E, F, whereas, for anything we know, E, F might coincide and the straight lines have three common points. The proof is therefore delusive. (Heath 196)
Heath believes that the better course is the one chosen by Euclid’s successors: namely, to add this fact to the Postulates:
It is however better to assume as a postulate the fact, inseparably connected with the idea of a straight line, that there exists only one straight line containing two given points, or, if two straight lines have two points in common, they coincide throughout. (Heath 196)
This is not the only ambiguous point in this postulate: Euclid does not explicitly say whether the two points must be in a given two-dimensional plane, rather than in three-dimensional space. The first six books of the Elements only deal with plane geometry, but Books 11-13 covers solid geometry (Books 7-10 deal with number theory, which Euclid studies from a geometrical perspective):
The last three books of the Elements cover solid geometry, and for those, the two points mentioned in the postulate may be any two points in space. Proposition 11.1 claims that if part of a line is contained in a plane, then the whole line is. In the books on plane geometry, it is implicitly assumed that the line AB joining A to B lies in the plane of discussion. (Joyce)
And that’s a good place to stop.
References
- Ernst Ferdinand August, Euclidis Elementa, Part 1, Theodor Trautwein, Berlin (1826)
- Henry Billingsley (translator), The Elements of Geometrie of the Most Auncient Philosopher Evclide of Megara, John Day, London (1582)
- Richard Fitzpatrick (translator), Euclid’s Elements of Geometry, University of Texas at Austin, Austin, TX (2008)
- Thomas Little Heath (translator & editor), The Thirteen Books of Euclid’s Elements, Second Edition, Dover Publications, New York (1956)
- Johan Ludvig Heiberg, Heinrich Menge, Euclidis Elementa edidit et Latine interpretatus est I. L. Heiberg, Volumes 1-5, B G Teubner Verlag, Leipzig (1883-1888)
- Henry George Liddell, Robert Scott, A Greek-English Lexicon, Eighth Edition, American Book Company, New York (1901)
- Glenn Raymond Morrow (translator), Proclus: A Commentary on the First Book of Euclid’s Elements, Princeton University Press, Princeton, NJ (1970)
- François Peyrard, Les Œuvres d’Euclide, en Grec, en Latin et en Français, Volumes 1-3, Charles-Frobert Patris, Paris (1814, 1816, 1818)
Online Resources
