Andrew Wiles and the Last Theorem

ANDREW WILES AND THE LAST THEOREM

INTRODUCTION

In the summer of 1991, an English mathematician achieved an ambition he had stuck at since childhood, which was to show that Fermat's Last Theorem can indeed be demonstrated as valid beyond all logical doubt. When you bare in mind that this was a challenge that had gotten the better of the most brilliant minds of the past three hundred years and that there was no real evidence that such a theorem was possible beyond Fermat's boast, you can appreciate what an achievement it was to persist for so long in trying to meet a challenge, and then ultimately succeed.

(Andrew Wiles)

EARLY YEARS

Wiles had first come across Fermat's Last Theorem in 1963 when he was ten years old. While visiting his town's library he found a book by one Eric Temple Bell called 'The Last Problem', which was all about the challenge that the French mathematician had set. Wiles was already keenly interested in maths and loved solving puzzles and he was soon gripped with the urge to become the one who would find the missing theorem. "Here was a problem that I, a ten-year-old, could understand and I knew from that moment that I would never let it go. I had to solve it".

As he continued to grow toward adulthood, Wiles developed his mathematical skills and learned all he could about the branches of maths that had been developed in the 20th century, hoping that these would provide him with a way of cracking the puzzle that had not been available to mathematicians who had come before. The theorem, however, remained as illusive as ever.

ELLIPTIC CURVES

Still, it was hardly wasted effort, because his determination to learn higher mathematics secured Wiles a place at Cambridge. He joined in Emmanuel College in 1975 and his supervisor was John Coates. It was Coates who suggested an area of study that would ultimately play a part in enabling Wiles to prove the Last Theorem.

Coates decided that Wiles should study a branch of mathematics known as 'elliptic curves'. These were so-named not because they were ellipses or curved but rather because they were equations that had been used to measure the perimeters of ellipses and the lengths of planetary orbits. When he joined Cambridge, Wiles had felt the need to temporarily abandon his quest to find the missing theorem. "The problem with working on Fermat was that you could spend years getting nowhere", he explained in the book 'Fermat's Last Theorem'. But actually elliptic curves were not entirely removed from his life's pursuit. For one thing, they lay down a challenge somewhat similar to Fermat's equations, which is to figure out if they have whole number solutions and how many solutions exist if so. Also, Fermat himself had discovered the proof that one elliptic equation has only one set of whole number solutions.

MODULAR FORMS AND THE TANIYAMA-SHIMURA CONJECTURE

In fact, elliptic curves would turn out to be more relevant to Fermat's Last Theorem than most people realised at the time. But in 1954, over in Japan, a pair of maths students at the university of Tokyo had made a startling discovery related to elliptic curves that would ultimately help Wiles prove Fermat's Last Theorem.

While at the University of Tokyo, Goro Shimura required a copy of Mathematische Ammalen, Vol 24, because it contained information necessary to help him with some fiendishly difficult calculations. But the library's copy had already been borrowed and the person who got there first was Yutaka Taniyama. After politely inquiring when Taniyama would be finished with the copy, Shimura learned that he was working on the same problem. The two decided to work together.

Taniyama and Shimura became fascinated by a branch of maths known as 'modular forms'. Not many people have heard of modular forms but they are actually considered one of the five fundamental operations of mathematics (the other four being the much more familiar addition, subtraction, multiplication, and division).

Modular forms are equations with an incredibly high level of symmetry. We say an object has symmetry when it can be transformed in some way and yet remain the same. Most objects we are familiar with have only limited symmetry. A white ball, for instance, can be rotated and still look the same so it as 'rotational symmetry'. But if you were to move the ball from left to right then somebody else who knew the ball's prior position would notice it had changed position. So it does not have translational symmetry. When it comes to modular forms, however, they have a level of symmetry beyond anything you are familiar with. It is pretty hard to convey this symmetry, however, because modular forms exist in a four-dimensional space known as hyperbolic space. As such, while you can write down the coordinates of such an object it is not possible to imagine what it looks like. The closest anyone has got to drawing a modular form is Maurice Escher, whose 'Circle Limit IV' is a two-dimensional approximation of a modular form.

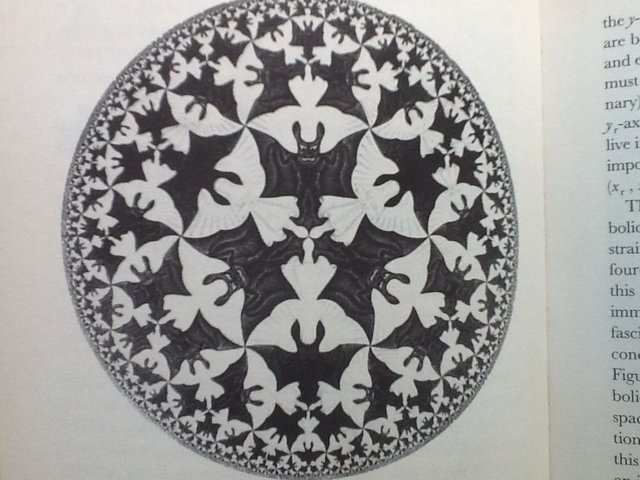
(Circle Limit IV)

For the purposes of this story, there is actually not much need to know anything about modular forms other than that such a thing exists and that, for the longest time, they were considered to have nothing to do with elliptic curves. If there is such a thing as the 'universe of mathematics', then conventional wisdom held that elliptic curves and modular forms lived in completely different regions and there was not even the remotest link between them.

But then, Taniyama and Shimura noticed a most curious thing. They came across a particular modular form that appeared to be very similar to a particular elliptic curve. Moreover, they discovered more modular forms that appeared to have an equivalent elliptic curve. As the pair continued to find modular forms that were related to elliptic curves, they began to suspect that every modular form might in fact be an elliptic curve in disguise. This hypothesis that 'for every modular form there is an elliptic curve' became known as the Taniyama-Shimura Conjecture.

However, this conjecture could not be proved by demonstrating that some particular modular forms were related to some particular elliptic curves, even if you had provided millions or even billions of examples consistent with that hypothesis. This is because there are infinitely many such equations and it would only take one modular form that cannot be related to an elliptic curve to invalidate the conjecture. Therefore, as is always the case in maths, a theorem had to be written that would demonstrate beyond all logical doubt that there is no such thing as a modular form that is unrelated to an elliptic curve.

THE KEY TO FERMAT'S LAST THEOREM

The story now turns to a small town in Germany called Olberwolfach. There, in 1984, a group of number theorists gathered together in order to discuss elliptic curves and whatever progress had been made in proving the Taniyama-Shimura Conjecture. One of the speakers, a man called Gerhard Frey, made a claim that would ultimately help Wiles in his quest. What he did was to transform Fermat's equation 'X^2+Y^2=Z^2 into an elliptic equation. However, this elliptic equation could only exist depending on certain assumptions being true. In particular, the elliptic equation Frey wrote down was so strange it could not possibly be related to any modular form. Therefore, Frey was able to show that the Taniyama-Shimura conjecture was the key to proving Fermat's Last Theorem because (as explained by Simon Singh)

"(1) If the Taniyama-Shimura Conjecture can be proved true, then every elliptic equation must be modular.

(2) If every elliptic equation must be modular, then the Frey elliptic equation is forbidden to exist.

(3) If the Frey Elliptic equation does not exist, then there can be solutions to Fermat's equation.

(4) Therefore Fermat's Last Theorem is true".

So, anyone who could prove the Taniyama-Shimura Conjecture would automatically also go down in history as the person who proved Fermat's Last Theorem. In the summer of 1991, Andrew Wiles succeeded in proving the Taniyama-Shimura Conjecture and, as a consequence, also became the man who succeeded where all previous generations of mathematicians stretching back over the past three hundred years had failed: He proved Fermat was correct to assert that there are no whole number solutions to X^n+Y^n=Z^n where n is any number higher than two. Wiles's proof is an astonishingly complex work that runs to over 130 pages and includes such obscure branches of mathematics as the Kolyvagin-Flach method, Galois groups, and the aforementioned Taniyama-Shimura conjecture which is concerned with elliptic curves and modular forms.

WILES' ACHIEVEMENT

As a non-mathematician, Wiles's paper proving the Taniyama-Shimura conjecture is completely incomprehensible to me. It may well lay claim to being one of the most complex logical arguments ever written by a human being. But I can still appreciate what an achievement it was to have come across a puzzle and to persist in trying to solve it for years on end, never giving up hope even though centuries of brilliant minds had failed. Few of us can expect to develop the mathematical skills necessary to match that of Andrew Wiles, but we can all learn something from his determination. After all, hard-won success comes to those with the mental discipline not to quit.

REFERENCES

"Fermat's Last Theorem' by Simon Singh

BBC Horizon documentary on Andrew Wiles