It seems paradoxical, or contradictory, to refer that within the scope of mathematics its most representative expression, the number, may have something irrational. How is it that the mathematical formality, that science full of accuracy and precision may have something to do with irrationality, that is, how is that irrational numbers.

link

Everything could be expressed through numbers

Well, everything is therefore an event that occurred more than 2,500 years ago, there in the fifth century BC. C, when that great discovery occurred that represented something like "The Crisis of the Irrationals".

link

Why would the emergence, or discovery, of that new type of number represent a crisis? Well, for that time, I repeat more than 2500 years ago, in full force of the Pythagorean school, and its doctrine, there were only whole numbers and fractions, the latter as a representation of the relations between the first, the integers. Furthermore, these numbers, for that time known, and handled, were only positive since the civilizations of antiquity did not know the negative numbers.

link

Then, only with the integers, and the fractions, everything related to the world and its harmony could be represented and expressed. However, there was a fact that completely transformed the previously known, and dominated, by those who worked most mathematically for the time, the Pythagoreans, and for whom "everything" could be expressed through numbers.

The crisis

Well, there was an event that went out of the way EVERYTHING! It can be represented through numbers.

In this context, it can be represented with numbers, we can place a square, and we can do it with the simplest of all, whose side is equal to the unit.

link

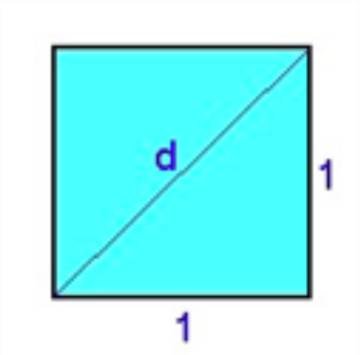
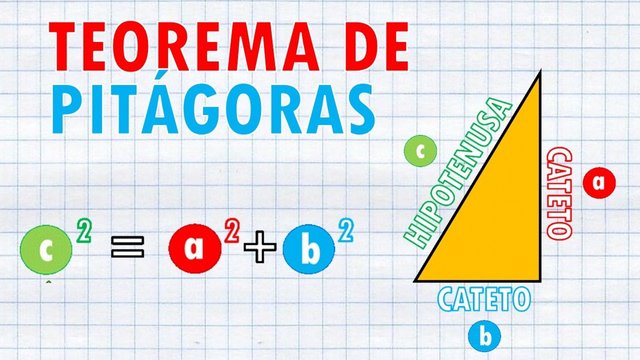
Regarding a figure like the one referred to, a mathematical model was already established, the Pythagorean Theorem, which allowed to establish the relationships between the sides of the figure in question. What the theorem affirmed: "The square of the hypotenuse, (in the square we are dealing with is the diagonal) is equal to the sum of the squares of the other two sides"

link

Then, returning to our square of side 1, it turns out that:

The square on the diagonal is equal to 2 (that is, the diagonal "d" was equal to the square root of 2)

link

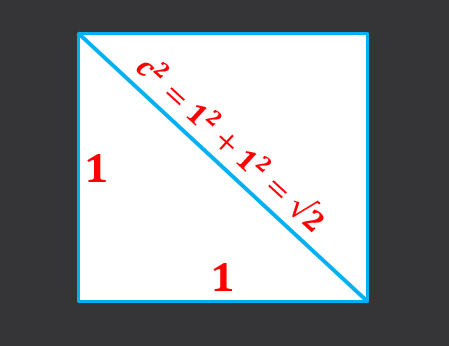
It was presented here, a kind of missing link, the length of the diagonal is a number whose square is equal to 2!
The Pythagoreans then asked themselves What is that number?. No number seemed to fit the situation, no number was ideal, that seemed irrational, because there were no numbers to express some magnitudes, so they were considered as inexpressible, as Irrational numbers!

For reflection

Look closely at the figure again.

link

The side of the square can be seen, the diagonal of the square can be seen and, nevertheless, incommensurability arises between them, that is, the diagonal can not be measured from the side. This is what the Pythagoreans demonstrated *** "If a number represents the side of a square, no other number can represent its diagonal" ***. That is, they showed that there can not be a rational number whose square is 2.

…This story will continue!!