27-10-2024 - Education - Geometry - Matrices exercises [EN]-[IT]

---

~~~ La versione in italiano inizia subito dopo la versione in inglese ~~~

---

[ENGLISH]
27-10-2024 - Education - Geometry - Matrices exercises [EN]-[IT]
With this post I would like to provide some brief notions about the technical topic mentioned in the subject.
The context in which we operate is that of analytical geometry
(code notes: MOD-89)

Matrix exercises
In this article I show some exercises with matrices, but I will not explain in detail what a matrix is ​​assuming that it is a topic already known.

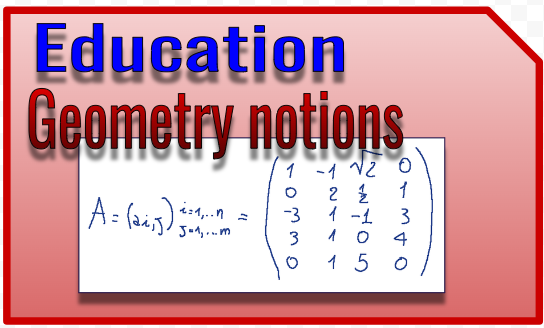
Let's consider the following matrix

Entries of a matrix
In analytical geometry, the entries of a matrix are the individual elements that make up the matrix, positioned in the various rows and columns. Each entry is identified by two indices that indicate its precise position within the matrix: the first index represents the row, while the second represents the column.

For example, if we have a matrix A with dimensions m x n, its entries are indicated by aij
Where:
m = number of rows
i = the index of the row
𝑛 = number of columns
j = the index of the column

For example, entry a11 is the entry that is found in the first row and in the first column

Taking into consideration the matrix of the exercise we can say that the entries (2,3). (1,4), (4,1) and (5,2) are as follows:
(2,3) = a23 = ½
(1,4) = a14 = 0
(4,1) = a41 = 3
(5,2) = a52 = 1

What is the fifth row of the matrix?
The fifth row of matrix A is
( 0 1 5 0 )

What is the third column of the matrix?
The third column of matrix A is

Conclusions
In geometry matrices are used to:
-Represent and calculate geometric transformations
-Solve systems of equations linear
-Representing and manipulating data
Matrices provide a powerful tool for solving geometric problems.

Question
When you took geometry in school, did your teacher also teach you matrices?

---

---

[ITALIAN]
27-10-2024 - Education - Geometria - Esercizi con le matrici [EN]-[IT]
Con questo post vorrei fornire alcune brevi nozioni a riguardo dell’argomento tecnico citato in oggetto.
Il contesto in cui operiamo è quello della geometria analitica
(code notes: MOD-89)

Esercizi con le matrici
In questo articolo mostro alcuni esercizi con le matrici, ma non andrò a spiegare nel dettaglio cosa è una matrice supponendo che sia un argomento già conosciuto.

Prendiamo in considerazione la seguente matrice

Entrate di una matrice
In geometria analitica, le entrate di una matrice sono gli elementi individuali che compongono la matrice, posizionati nelle varie righe e colonne. Ogni entrata viene identificata da due indici che indicano la sua posizione precisa all'interno della matrice: il primo indice rappresenta la riga, mentre il secondo rappresenta la colonna.

Se per esempio abbiamo una matrice A con dimensioni m x n le sue entrate vengono indicate con aij
Dove:
m = numero righe
i = l’indice della riga
𝑛 = numero colonne
j = l’indice della colonna

Per esempio l'entrata a11 è l’entrata che si trova nella prima riga e nella prima colonna

Prendendo in considerazione la matrice dell’esercizio possiamo dire che le entrate (2,3). (1,4), (4,1) e (5,2) sono le seguenti:
(2,3) = a23 = ½
(1,4) = a14 = 0
(4,1) = a41 = 3
(5,2) = a52 = 1

Qual è la quinta riga della matrice?
La quinta riga della matrice A è
( 0 1 5 0 )

Qual è la terza colonna della matrice?
La terza colonna della matrice A è

Conclusioni
In geometria le matrici servono per:
-Rappresentare e calcolare trasformazioni geometriche
-Risolvere sistemi di equazioni lineari
-Rappresentare e manipolare dati
Le matrici forniscono uno strumento potente per risolvere problemi geometrici.

Domanda
Quando a scuola avete fatto geometria, il professore vi ha insegnato anche le matrici?

THE END