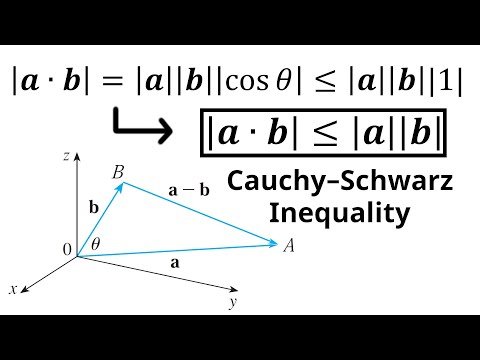
In this video I show that the absolute value of the dot product has a maximum value of the lengths of each vector multiplied by each other. This relation is known as the Cauchy-Schwarz Inequality when written in its most basic form using absolute values and the dot product.
Since the geometric formula for the dot product is equal to the magnitudes (or norms) of each vector multiplied by each other and multiplied to the cosine of the angle between them, then the maximum value of the dot product is when the cosine is at a maximum. And the absolute maximum value of cosine is 1, thus the geometric formula simplifies to just the Cauchy-Schwarz Inequality.
Time stamps:
- Question 22: Absolute value of dot product is less than multiplication of each vector length: 0:00
- Absolute value of cosine function is less than 1: 0:50
- This is known as the special case of the Cauchy-Schwarz Inequality: 2:00
Full video and playlists:
- Full video and playlist: https://www.youtube.com/playlist?list=PLai3U8-WIK0GiYS2qR98dzUjsx-0PRPbC
- Notes: https://peakd.com/hive-128780/@mes/vectors-geometry-true-false-quiz
- Vectors and the Geometry of Space: https://www.youtube.com/playlist?list=PLai3U8-WIK0FjJpwnxwdrOR7L8Ul8VZoZ .
Become a MES Super Fan! https://www.youtube.com/channel/UCUUBq1GPBvvGNz7dpgO14Ow/join
DONATE! ʕ •ᴥ•ʔ https://mes.fm/donate
SUBSCRIBE via EMAIL: https://mes.fm/subscribe
MES Links: https://mes.fm/links
MES Truth: https://mes.fm/truth
Official Website: https://MES.fm
Hive: https://peakd.com/@mes
Email me: [email protected]
Free Calculators: https://mes.fm/calculators
BMI Calculator: https://bmicalculator.mes.fm
Grade Calculator: https://gradecalculator.mes.fm
Mortgage Calculator: https://mortgagecalculator.mes.fm
Percentage Calculator: https://percentagecalculator.mes.fm
Free Online Tools: https://mes.fm/tools
iPhone and Android Apps: https://mes.fm/mobile-apps
▶️ DTube
Getting in those math reps!
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit