By following previous post , in this post we will show the rest terms are equivalent.
Recall that
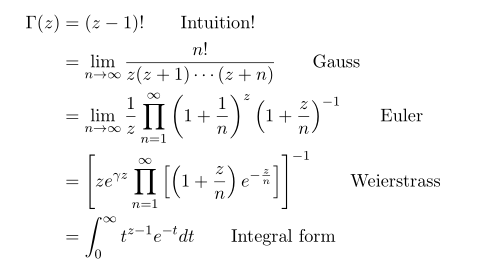
and in the last posting we have shown that expression of factorial, Gauss and Euler are equivalent to each other. In this post we will extend this to Weierstrass and integral form.
Weierstrass
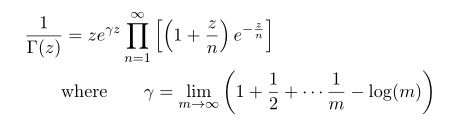
Thhis expression contains Euler constant gamma, so this definition is powerful when we encounter di-gamma and poly-gamma function.
For this Weierstrass gamma function we will show that this expression is equivalent to Euler form.
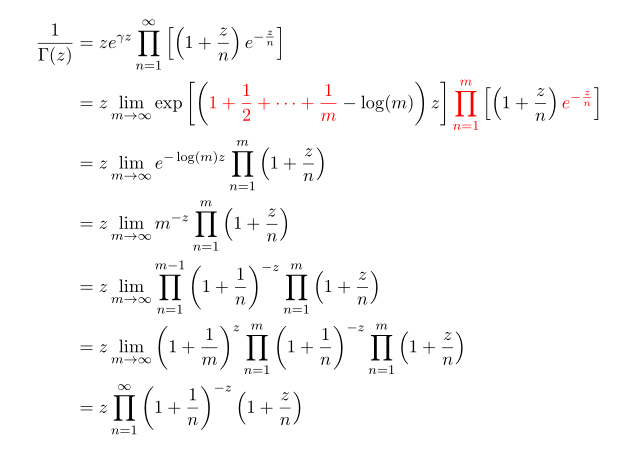
In the process we used
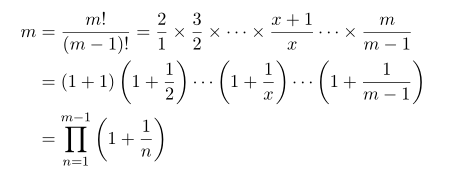
Integral form
This is the most well-known expression for gamma function.

For Re(z)>0, by integration by parts we can easily see that this expression is equivalent to the Factorial.
But this is not funny, so by little deformation check the equivalence to other version of gamma function.
First slightly deform the gamma function as
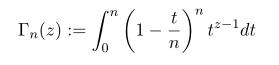
of course this is well-defined on Re(z)>0
By Integration by parts

take n to infinity we can see that this function is actually the gamma function.
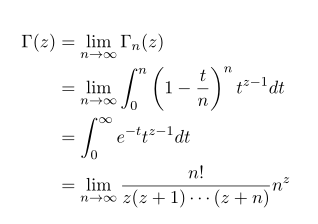
Thus the integral form of gamma function is equivlanet to the Gauss version of gamma function.
저도 지금 수학에 관한 무언가를 포스팅해볼까 하는데 조금 더 중고딩에 도움되는 것으로 해볼까 합니다. 이미 전문적인것은 beoped님이 잘해주시고 계시니까요 ^^
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
ㅎㅎ 중고교 과정에서도 괜찮은 주제들이 많이 있는 것 같아요 ㅎㅎ
앞으로 올라오게 될 포스팅 기대할게요 ㅎㅎ
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit