For this time using Fourier expansion, we want to compute zeta 4.
To do that first briefly mention Fourier expansion of function f(x)
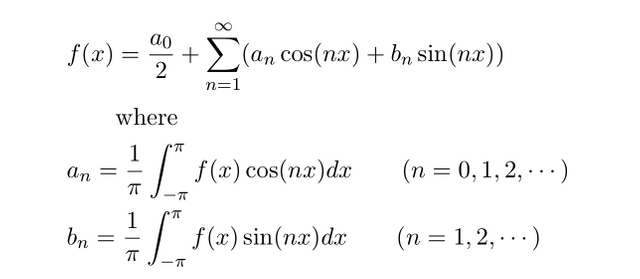
This technique is freuqently used in many science and engineering fields. From the shape of function f(x), we have some special properties. For example when f(x) is even, from parity, b_n=0, and for f(x) is odd, again from parity, a_n=0, and so on.
To compute zeta 4, our choice of f(x) is

which is even function.
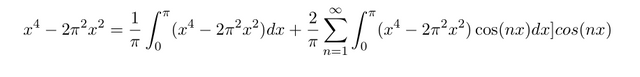
Note that the first few terms are integration over polynomial on x, so we can easily compute. Then how about the later terms?
Leibniz integral Rule
Using Leibniz integral Rule we can compute integral easily.
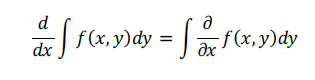
For example
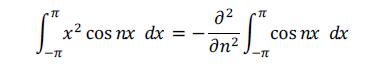
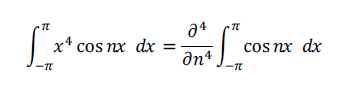
Generalized

Now using this equation, by plugging we have
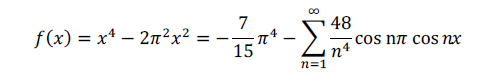

Plugging

and re-ordering we have

ohhh muy interesante la funsión de Fourier
Saludos :)
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
It is very difficult for me, athletes)
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit