Homer threw a baseball to Bart. Like that:

Notice that at the moment in time depicted on the image, the ball is located at a certain fixed position in space. While it is being in that fixed position, it is certainly not moving. Because, as we know, an object in a fixed position must be fixed, by definition, right?
But this logic must then be true for any timepoint. Indeed, no matter when you look at the ball, it must always be "frozen" at a certain fixed position in space.
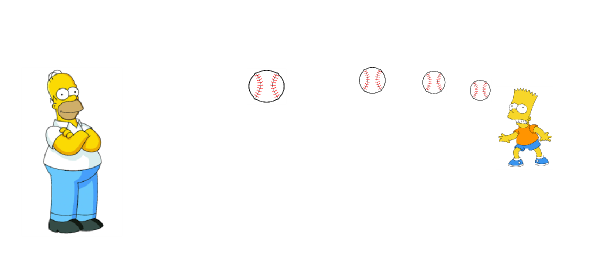
Now, if the ball is "frozen" at all points in time, there are no observable moments when it moves. We must therefore conclude that the ball, in fact, never moves at all!
This is the third famous paradox of Zeno, commonly known as the "Arrow paradox" (because in the original story Achilles was shooting an arrow rather than throwing a baseball, of course). While the first two Zeno's paradoxes are mathematical sophisms, which can easily be resolved with some proper calculus, this one is very different.
Rather than being just a mathematical curiosity, it is primarily a philosophical question about the nature of time. What is time if not a collection of fixed snapshots of the universe we observe around us? And if it is, how and why are these snapshots changing one into another so that things seem to move?
For Bart and Homer in the cartoon, we actually know the answer: it is the video rendering algorithm, which continuously replaces each frame with the next one. If Bart and Homer were characters of a computer game instead, the state of their world would be updated by a CPU running the program. But what about us and the real world? Is our time discrete or continuous? Are we all just part of an exciting movie with someone changing frames? Is it all a big computer simulation? How and why do things change around us? What is time, after all?
Take some time to think about it.
For other sophisms check out this list.
The smallest interval is Planck time, which is the time required for light to travel a Planck unit in vacuum. I suspect if you go smaller than this, the object is in multiple positions.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
True, yet the existence of the Planck time constant does not really let us claim that time is necessarily quantized (here is a nice argument on this).
On the other hand, this does not mean it is impossible that the universe is a big computer with a discrete timestep either. Unfortunately, though, such a hypothesis is most probably unfalsifiable and hence outside the reach of scientific method.
From a practical perspective it makes most sense to consider time continuous simply because that's how it is postulated in most (probably all) physical theories today and these theories works totally fine.
Yet it is this continuous time assumption where the arrow paradox looks most paradoxical.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Hi " " Welcome to Steemit.
I can Follow You.
You can follow me.Thnx
https://steemit.com/@muhmmadjamshed/transfers
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
A bot like that seems rather uncool to me. A bot which fails to find out the name of the user it is posting to is twice as pathetic.
In general, what's the deal with the random "follow me" requests at all? Does it pay to have many random irrelevant followers?
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Congratulations @konstantint! You have completed some achievement on Steemit and have been rewarded with new badge(s) :
Click on any badge to view your own Board of Honor on SteemitBoard.
For more information about SteemitBoard, click here
If you no longer want to receive notifications, reply to this comment with the word
STOPDownvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit