What are the main exponent rules?
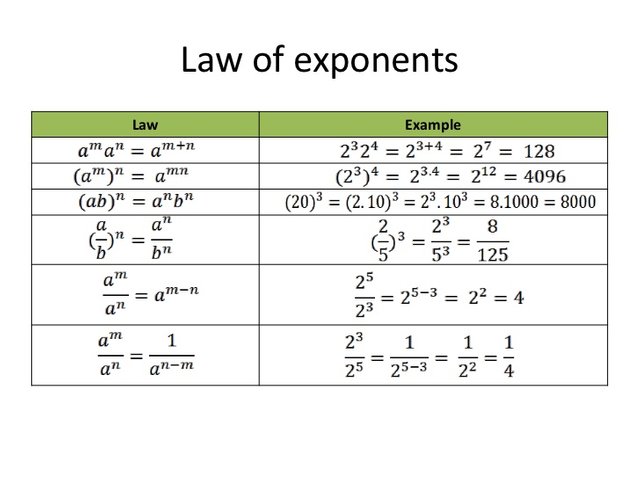
Rules of Exponents: There are 4 basic exponent rules. Students in grade 7 and beyond need to be familiar with these rules and should be able to apply these rules when dealing with problems involving exponents.
Zero Exponent Rule or Power Zero Rule: This is a very very basic rule to remember as most often overlooked by students and which cost them lot of marks in their math quizzes and exams. The rule is that "If any number raised to the power of zero then its value is equal to one".
For example; consider the problem, 2º which is read as two raised to the power zero. The answer is equal to 1 as shown below:
2º = 1
Below are more example on zero exponent law
5º = 1
9º = 1
Or in case of algebra (5xy)º = 1
Make sure you do it right when there involves negative number as the base. Look at the example below to understand how the negative sign can change the answer when parenthesis are used and when not used:
(-3)º = 1 but -3º = - 1
2. Law of Exponent Multiplication: In case there are two exponents being multiplied and their bases are the same then we can add the powers (exponents) to get the new power (exponent) and base remains the same. For example;
5² x 5 {we can write it as follows as 5 can be written as 5¹}
= 5² x 5¹ { add the powers 2 and 1 to get the new power as 3 and base remains the same}
= 5³
3. Law of Exponent Division: If we divide two exponential terms and their bases are same then we subtract the power of the denominator from the power of the numerator to get the new power and base remains the same. For example;
3³/3²
= 3¹ {But 3¹ is same as 3 hence we can write the final answer as shown below}
= 3
4. Exponent of an exponent: There is another rule involving exponents which is exponent of another exponents. These problems look like (3³)² and to solve it just multiply both the exponents to get the new exponent and base remains the same. We can solve it as follows
(3³)² = 3³˙² = 3^6 (which is 3 raised to the power of 6)
Below is a practice sheet to practice all the laws of exponents

Hi there....I have done all these laws with my kids now during this last month. They struggle terribly with the negative exponent rules. Especially where a variable with a negative exponent is divided with a variable with a negative exponent. Any chance of doing a post only on this? Thanks this is great work!
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
I will love to do that next time giantbear :)) Actually start work on that right now.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
I will appreciate that very much. It is so difficult to explain that to students and frankly I confuse myself...lol
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
I will do two posts on negative exponents. One covering the basic concepts and other one on solving complex problems involving negative exponents and variables. Will also post couple practice sheets so that students should we able to practice what they have learned.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Thank you so much Manjit. You are wonderful!!!!
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
It's great to have a math teacher here on Steemit.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
It certainly is. I much prefer using @mathworksheets pdf's in my class than from any other website.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Thanks for your true support kus-nee and ginatbear:))
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
It would be nice to have more texts like this. Keep going, Manjit!
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
I will keep doing this as there is a lot to share. Thanks a lot for your comments lighteye.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit