Rules To Multiply & Divide Integers

 Rules to multiply integers:  

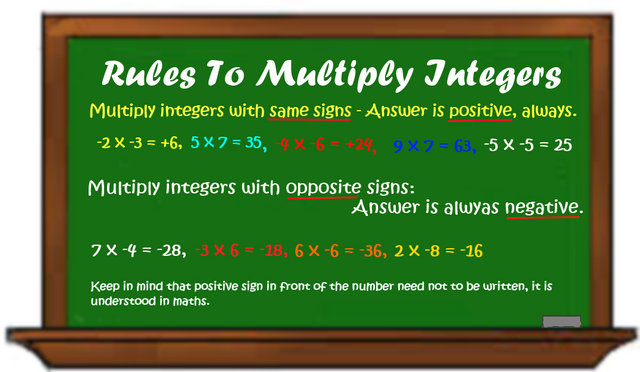
To multiply integers use your times tables skills to multiply the numbers. To decide the sign of the answer, if the numbers you multiplied have the same signs (both either positive or negative), the answer is always positive. 

Let’s clear this by doing the following examples to multiply integers; 3 x 6 = 18  

Numbers, 3 and 6 got the same (positive) signs, so their multiplication is positive. 

Now let's do; - 3 x – 6 = 18 

Again numbers, 3 and 6 got the same (negative) signs; therefore their multiplication is positive again.    

For multiplying negative signed numbers, brackets are often used and – 3 x – 6 can be written as - 3 x (- 6) too or can be written as – 3 (- 6) or as (- 3) (- 6).  

Notice that in math, if there is just an open bracket between two numbers, it means those numbers are getting multiplied with each other. In other words, the numbers have a "multiply (times) sign" hidden between them.

Further, if the signs of the numbers getting multiplied are different, the answer is always negative. For example consider the numbers 3 and 6 again, but with different signs, - 3 x 6 = -18 

or – 3 (6) = - 18   or it can be written as (- 3) (6) = - 18  

Numbers, 3 and 6 got the opposite signs; therefore their multiplication is negative.   

3 x – 6 = - 18   OR    3 (- 6) = - 18     OR     (3) (-6) = - 18 

Again numbers, 3 and 6 got the opposite signs; therefore their multiplication is negative.   

Rules to Divide Integers: 

Rules to divide integers are the same as rules for multiplying them. If an integer divides another integer with the same sign; the quotient (answer) is always positive. 

For example; 20 ÷ 5 = 4   or

(- 20) ÷ (- 5) = 4   OR  this can be written as -20 ÷ -5 = 4   

If the integers got the opposite signs their quotient is always negative. For example; -20 ÷ 5 = - 4    OR   20 ÷ (-5) = - 4     

Summary: · When multiplying two integers with the same signs, the answer is always positive. · When multiplying two integers with different signs, the answer is always negative. · 

When an integer divides another integer with the same sign, the quotient is always positive.

When an integer divides another integer with the different sign, the answer is always negative.   

Note: Know the difference! 

This is the last presentation on integers and we have explored all the four operations with integers. Be careful not to mix all the rules with each other. I often notice that some of my students have difficulty in applying same sign rules on addition and multiplication as both operations have the same sign rule, but the the application of the rule for the both operations is not same. 

For example; - 10 – 4 = -14 

Notice that there is no multiplication sign or parenthesis, which means, you look at the signs first. The numbers got same signs and you know that same signs add and keep. Therefore, – 10 and – 4 got added to get  – 14 as the answer.  

Same numbers can make another problem as shown below; 

-10 x – 4     or     -10 (-4)      or      (-10) (-4)    and all = 40 

All of the above forms represent the same problem which is multiplying -10 to -4 (-10 times -4), which you can solve easily, 10x4 is 40 and both numbers got the same signs therefore answer 40 is positive.   

Conclusion:  Carefully decide the operation you are applying to solve an integer problem. In above example, in first case -10 and -4 gave the answer -14 and in the second case they gave the answer 40. 

To understand difference if you need to multiply or add the integers I suggest to do a lot of practice exercises on addition and multiplication of integers. 

Following are more examples to clear you a bit more on this issue; 

4 + 5 = 9 which can also be written as 4 - - 5= 9 (it is the addition of integers)

But (4) (5) = 20 which can also be written as 4 x 5 = 20   (it is the multiplication of integers)

- 4 – 5 = -9 which can also be written as -4 + (-5) = - 9 

-4 (-5) = 20 which can also be written as -4 x -5 = 20 

Now 4 – 5 = - 1 or 4 + (-5) = -1  (which is integer subtraction)

But 4 x -5 = - 20 or 4 (-5) = -20  (which is integer multiplication)

Also - 4 + 5 = 1 or – 4 – (-5) = 1 

- 4 x 5 = - 20 or (-4) (5) = - 20 

Notice that, where there is no sign between two brackets, or between a number and a bracket, or multiplication (times) sign between the two numbers; MULTIPLY the NUMBERS. If the numbers you multiplied got the SAME SIGNS the ANSWER is always POSITIVE. If the NUMBERS you multiplied got the OPPOSITE SIGNS, the ANSWER is always NEGATIVE.