“When I was ten, my father told me about Euclid's proof [of the infinitude of primes], and I was hooked.” -Paul Erdos
“My brain is open.” -Paul Erdos

Paul Erdos was a Hungarian mathematician who lived from March 1913 to September 1996. Dr. Erdos loved elementary number theory, combinatorics, analysis, set theory, and graph theory. He was an extremely prolific mathematician and a world traveler of indefatigable energy who lived out of two small suitcases. Paul Erdos lived an extremely unconventional life, not having a fixed home and traveling from one math conference to the next while staying with different colleagues who gave him shelter; plus he was highly eccentric with various stories about his unusual behavior (and his strange personal vocabulary) becoming legendary. Erdos ended up authoring over 1500 math papers with many different collaborators (over 500) around the world.
Paul Erdos's first big result in number theory came when he was only 17 years old – he found an elementary proof of Bertrand's postulate which states that there is always a prime between n and 2n. Erdos liked working on problems that were easy to explain and understand, yet exceedingly difficult to prove. Rather than building extensive theories, Erdos enjoyed solving problems and his primary focus was to prove them in a simple, elegant way, hoping to provide keen insight and overall understanding rather than just listing a collection of rigorous steps to provide a stale formal proof.
(I first became aware of Paul Erdos from reading Paul Hoffman's excellent book, The Man Who Loved Only Numbers: The Story of Paul Erdos and the Search for Mathematical Truth, which I highly recommend (even though it does contain some errors).)
Notice the concrete prime at the beginning of this post, which spells out “Erdos” in its decimal expansion. Hopefully the name can be seen clearly by the reader. This prime is a tribute to the incredible Hungarian mathematician Paul Erdos. The Erdos prime above is an example of what I like to call “concrete mathematics” since the concept (in my opinion) is very similar to concrete poetry (poems in which the typographical arrangement of symbols or words plays a direct role in conveying the “meaning” of a poem). But where concrete math differs from concrete poetry is that it deals primarily with classes of numbers (primes, squares, triangulars, harshads, etc.) which possess a certain visual component when the digits are arranged in a certain way – i.e. the digits are “formatted” in rows so that a word, phrase, or image can be “pictured” in the decimal expansion. (Remember that the definition of a prime number is an integer without any divisors except for itself and one. For example, 2, 3, 5, 7, 11, 13, ... are all prime numbers.)
Notice the symbols: “[asterisk]10^218+1” at the very “bottom” of the Erdos prime given above. This “mathematical extension” if you will is absolutely necessary for the number to be prime, since basically I am treating the large main block of digits (ending with the long string of 1s) with “Erdos” spelled out in the decimal expansion as a constant (let's call it E) in a polynomial that I then run a search on to hopefully find a prime value for E[asterisk]10^n+1. Usually I prefer that the exponent value be small enough so I can simply list all the digits in one complete “block” and not have to resort to putting in the “[asterisk]10^n+x” value at the end, which I feel does not properly represent the prime since it slightly ruins the lack of simplicity and symmetry that can be achieved by listing all necessary digits in one large chunk. Adding the mathematical extension at the end causes the prime to lose a little of its special character and charm, but there's really nothing I can do about that – my job is to merely hunt for the specific concrete prime and accept whatever pops up.
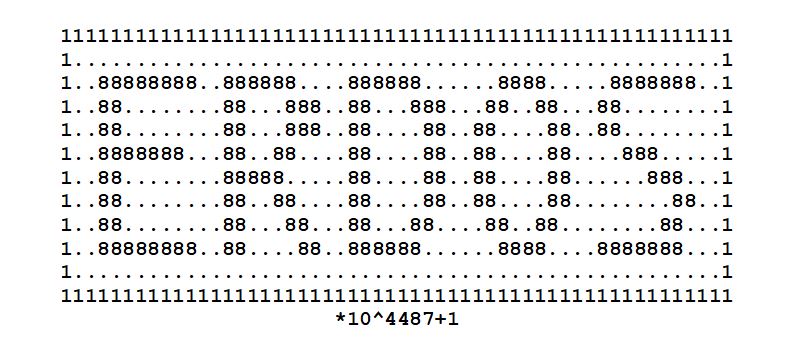
Usually the concrete primes I find will have more prime values associated with them than the main one listed at the beginning of this post. And for the Erdos prime this was also the case: others with the same Erdos decimal expansion but with different digits at the end are: E[asterisk]10^445+1; E[asterisk]10^583+1; E[asterisk]10^2110+1; and E[asterisk]10^3513+1; perhaps the reader would like to compute their decimal expansions and have fun formatting them like the Erdos prime given at the beginning of this post (and the one at the end.) Keep in mind, the formatting of the Erdos concrete prime is very important. Here is what it looks like in an unform-atted state:
11111111111111111111111111111111111111111111111111111110000000000000000000000000000000000000000000000000000110088888888008888880000888888000000888800000888888800110088000000008800088800880008880008800880008800000000110088000000008800088800880000880088000088008800000000110088888880008800880000880000880088000088000088800000110088000000008888800000880000880088000088000000888000110088000000008800880000880000880088000088000000008800110088000000008800088000880008800008800880000000088000110088888888008800008800888888000000888800008888888000110000000000000000000000000000000000000000000000000000111111111111111111111111111111111111111111111111111111100000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000000001
Not especially attractive now, is it? Yet it's still amazing to me that out of all those 8s, 0s, and 1s an actual “name” came be conjured up through simple formatting that represents one of the greatest mathematicians of all time.
Another Erdos prime is this one (notice the large “[asterisk]10^4487+1” extension at the end) except you will have to replace the periods below with zeros to make it a legitimate prime ready to be tested by your favorite prime-testing software:
Can you construct your own concrete Erdos decimal expansion (different from the one in this post) to find some Erdos concrete primes of your own? Can you find another form of number (perhaps square, harshad, or triangular) that has the name “Erdos” pictured somewhere in its digits?
Congratulations @zevi35711! You have completed some achievement on Steemit and have been rewarded with new badge(s) :
Click on any badge to view your own Board of Honor on SteemitBoard.
For more information about SteemitBoard, click here
If you no longer want to receive notifications, reply to this comment with the word
STOPDownvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Thanks for the award!
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Upvoted. Your article has been selected to appear in the next Math-Trail magazine.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Thank you! I will check out Math-Trail magazine.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Upvoted and followed.
This post reminds me of Tupper's self-referential formula in some sense.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Sounds interesting, I'll look it up.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit