
In this order of ideas, The Pythagorean School lasted approximately 150 years and during its existence hosted many great philosophers, and particularly mathematicians, among which are: Hippocrates of Chios, Theodore of Cyrene, Philolaus , Arquitas de Taranto and, of course, our guest of honor Hipaso.
Hipaso, was one of the first Pythagoreans within that School, was responsible for the "Acusmatic", that is, the candidates for initiation, while Pythagoras was the Head of the "Mathematicians", those already initiated.

In this context, Hipaso, the Head of the Acusmatics, was the creator of the Harmonic Media that would accompany the already known Arithmetic Mean and Geometric Media uniquely known before Hipaso introduced his.
The Arimetic Media
The arithmetic mean of two numbers a and c is known simply as the semi-sum of the numbers a and c. That is, the arithmetic mean between a and c, is another number b that is obtained by adding a with c and the result dividing it by 2. At the dedition level, sum and difference are used for arithmetic mean.
"The excess of the first number and relation to the second is the same as the excess of the second in relation to the third"

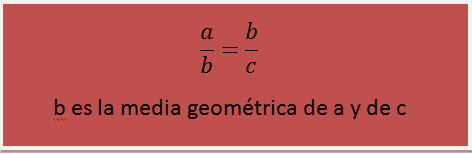
The Geometric Media
The geometric mean of two numbers a and c, relates multiplication and division. It is defined as follows:
"The first is to the second what the second is to the third"
Harmonic Media
The harmonic mean is somewhat more complicated, in its definition, than the two previous means.
For two numbers a and c, the harmonic mean between the two is another number c, such that: The first surpasses the second in a fraction of itself, while the second surpasses the third with the same fraction of the third "
So, for example, 4 is the harmonic measure of 6 and 3, since 6 exceeds 4 in 2, which is one third of 6, and 4 exceeds 3 in 1, which is one third of 3. Easy truth! !

Well friends up to this publication, in a next post we will address another episode, very interesting, in the life of our guest Hipaso de Metaponto.
Congratulations! This post has been upvoted from the communal account, @minnowsupport, by josmar2511 from the Minnow Support Project. It's a witness project run by aggroed, ausbitbank, teamsteem, someguy123, neoxian, followbtcnews, and netuoso. The goal is to help Steemit grow by supporting Minnows. Please find us at the Peace, Abundance, and Liberty Network (PALnet) Discord Channel. It's a completely public and open space to all members of the Steemit community who voluntarily choose to be there.
If you would like to delegate to the Minnow Support Project you can do so by clicking on the following links: 50SP, 100SP, 250SP, 500SP, 1000SP, 5000SP.
Be sure to leave at least 50SP undelegated on your account.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit