Source

We can classify numbers as follow:

Natural numbers:

• Numbers from 1 until infinity
• Natural numbers are all positive numbers

The set of natural numbers:

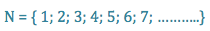
Whole numbers:

• Numbers from 0 until infinity
• Whole numbers are all positive

The set of whole numbers:

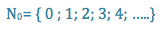
Integers:

• Integers are numbers that include all the positive and the negative whole numbers plus 0 (zero)

The set of integers:

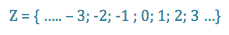
Not all numbers can be written as natural, whole numbers or integers.  Therefore the need for:

Rational numbers:

• Rational numbers are numbers that can be written in the form: 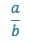 where b ≠ 0.
• These numbers include all the integers, common fractions, improper fractions, mixed numbers and decimal fractions- recurring and terminating decimals.
• Recurring decimals: Decimals that repeat endlessly in a specific pattern.  For example-
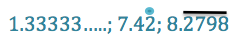
• Terminating decimals are decimals that end:
• The symbol we use for rational numbers is Q.

Irrational numbers:

• Irrational numbers cannot be written as
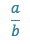
• Irrational numbers does not fall into one of the categories above.
• Irrational numbers includes decimals that do not terminate or repeat.
• This means that if these numbers are written as a decimal , it can only be represented by a decimal that continues indefinitely .
• For example:  
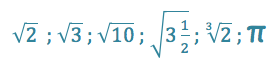
• The symbol that represents irrational numbers is Q'

Real numbers:

• Real numbers are rational + irrational numbers.
• The symbol that represents real numbers is R

Classification of numbers