MATHS TODAY||INDICES---the basics.

Ah ahh...

Today is going to be another day that we will refresh our memory on the basic mathematical operation that we must have forgotten, guess I have not seen, so far any article on maths...so I choose to take up the challenge, and I believe we will benefit from this in one way or the other. Welcome once again.

INDICES


Rings a bell right?

Indices is a word used to represent the power of a particular number in its multiplied state/form. Its is the plural of the word "index".

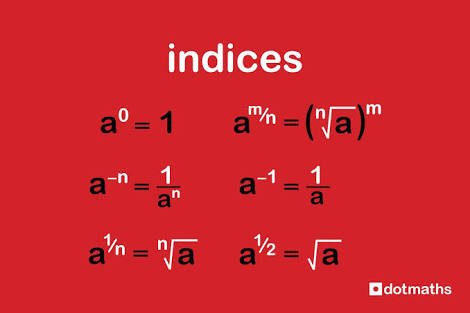
image source

In this article, I will remind us of the manipulations in indices, state the rules/laws which can be used to simplify expressions involving indices, and do some examples. Enjoy it with me as I proceed.

POWER

It is used to show that a quantity is repeatedly multiplied by itself.
Let's consider number 3 for example, power can be represented as;

3 x 3 x 3 x 3 = 3^4

OR

a × a × a × a = a^4

It can be read as ‘three to the power four’, because three(3) is written in four(4) places

N/B: '^' represents raised to power or a small number(index) at the top of the big number(also known as the base).

RULES OR LAWS OF INDICES

• RULE 1:
  

The first rule tells us that if we are multiplying expressions with the same base then we add the indices
together.

So, if we have;

a^m × a^n

we add the indices to get;

[a^m × a^n = a^m+n]

• RULE 2:
  

Suppose we have a^4 and want to raise it all to the power 3.

That is;

(a^4)^3

This means that;

a^4 × a^4 × a^4

Now our first rule tells us that we should add the indices together. So that is
a^12, But note also that 12 is 4 × 3. This suggests that if we have a^m all raised to the power n the
result is obtained by multiplying the two powers to get [a^m×n], or simply [a^mn].

• RULE 3:
  

This is usually known as the division rule.
Consider,

a^7 ÷ a^3 = a^7 ÷ a^3 =
a × a × a × a × a × a × a ÷
a × a × a

We can now begin dividing out the common factors of a. Three of the a’s at the top and the
three a’s at the bottom can be divided out, so we are now left with

a^4 ÷ 1

that is; a^4

The same answer is obtained by subtracting the indices,

that is, 7 − 3 = 4.

This leads us to the
third rule, that;

[a^m ÷ a^n = a^m−n].

• RULE 4:
  

Let’s have a look at a^3 divided by a^3.

We are dividing a quantity
by itself, so the answer has got to be 1.

a^3 ÷ a^3 = 1

Let’s do this invoking the 3rd rule; this tells us that to divide the two
quantities, we subtract the indices:

a^3 ÷ a^3 = a^3−3 = a^0

Oh, we have obtained a different answer. But, we have done the same calculation in two
different ways and have done it correctly in both ways. So we try to balance up the two different answers we've gotten, so that we have;

[a^0 = 1].

• RULE 5:

Let’s have a look at another division, but here, I will make the denominator bigger than the numerator.

Consider, a^3 divided by a^7.

a^3 ÷ a^7 = a × a × a ÷ a × a × a × a × a × a × a

Again, we can now begin dividing out the common factors of a. The 3 a’s at the top and three
of the a’s at the bottom can be divided out and we are now left with;

a^3 ÷ a^7 = 1 ÷ a × a × a × a = 1 ÷ a^4

By invoking our third rule and do the same calculation by subtracting the indices.

a^3 ÷ a^7 = a^3−7 = a^−4

Just like the later, we have done the same calculation in two different ways and correctly. So by balancing the two correct answers, we get;

[1 ÷ a^4 = a^−4] OR [a^−m = 1 ÷ am] OR [1 ÷ a^−m = a^m]

N/B: A negative sign in the index can be thought of as meaning ‘1 over’.

• RULE 6:

So far we been talking about integer powers(both positive and negative). What would we do if we had
a fraction for a power, like a^1÷2. To see how to deal with fractional powers consider the following:

We have two identical numbers multiplying together to give another number, as in;

7 × 7 = 49

Then we know that 7 is a square root of 49. That is, if

7^2 = 49 then 7 = square root of 49(49^1/2).

Now suppose we found that;

a^p × a^p = a

That is, when we multiplied a^p by itself we got the result a. This means that a^p must be a square
root of a.

However, in another way: a = a^1, and also that, from the first rule,

a^p × a^p = a^2p we see that if a^p × a^p = a then

a^2p = a^1

from which;

2p = 1(from index)

and so;

p = 1÷2

This shows that a^1/2 must be the square root of a. That is

[a^1/2 = square root of a(a^1/2)].

Also;

In cubed root, [a^1/3 = 3rd root of a].

The same goes for the fourth, fifth roots, etc.

Believe me that on my next article on Maths(indices), we'll look at some examples that obey these rules. But, until then, acquaint yourselves with the rules above.

Thanks for learning with me.

For more;

Follow and Upvote @dazzy.