
Mathematics is life, not everyone knows this. Ever wondered why it's made compulsory when you pass through school? You can't escape it because you perform some arithmetics in every day.
Today's tutorial will be on COMBINATION.
#Now, What is COMBINATION?
COMBINATION is the several possible ways in which a set or number of things can be selected or chosen.
Click here to read the tutorial on permutation because we will still use some formulae and simplification here which might new to you.
The keywords to note is that in permutation we are arranging while the combination is selecting.
In a permutation, an order is very important, that is ABC, ACB, BAC, BCA, etc are a different arrangement. But in COMBINATION, an order is irrelevant, for ABC, ACB, BAC, BCA, etc are the same selections.
Generally, the number of combination of selecting r objects from a distinct( same) objects is denoted by;
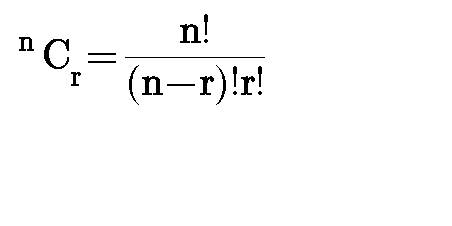
Where,
n= number of distinct objects
r= the number of objects to be selected from n
Let's try some examples,
Example 1: In how ways can three men be chosen from eight men?
Having read the question, you can see that we are to select not arranging. Do you agree with that? If no, read the question again.
Solution
n= 8
r= 3
Substituting values of n and r in the formula
We will have,
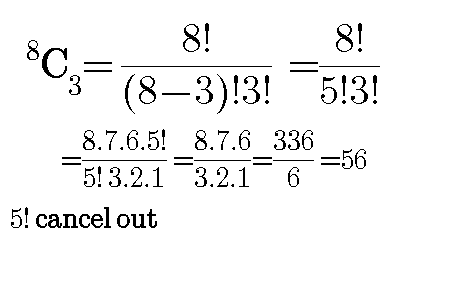
The number of ways to select 3 men from 8 men is 56ways.
Do you understand what we just did? if no, go through the question and solution again.
Example 2: In how many ways can three men and two women can be chosen from six mean and four women?
Solution
There are 6 men out of which 3 will be chosen. This can be done in 6C3 ways. Also, there are 4 women out of which 2 will be chosen and it can be done in 4C2 ways.
Hence the number of ways in which 3 men and 2 women can be chosen from 6 men and four women are,
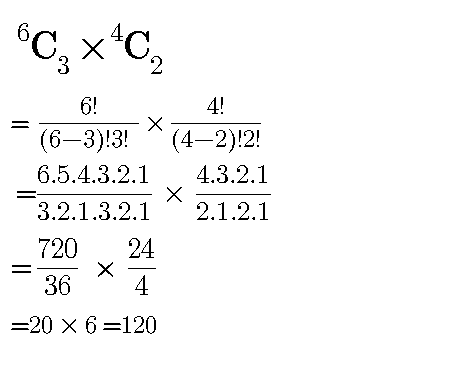
Therefore, there are 120 ways of choosing three men and two women from six men and four women.
Is it clear?
Example 3: A party of 4 or more to be selected from 10 persons, how many ways can this be done?
Solution
Having read the question, what is the number of persons to be selected from 10 persons?
You can see that 4 or more was stated in the question.
The number to be selected is 4, 5, 6, 7, 8, 9, 10
n= 10
r=4,5,6,7,8,9,10
i.e
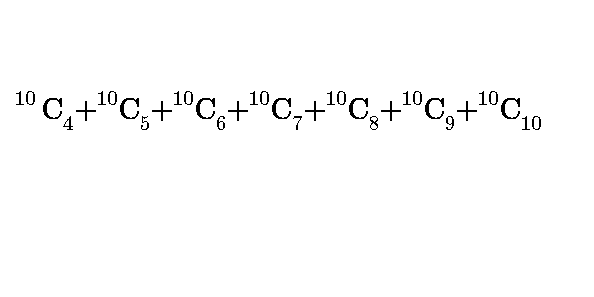
By simplifying the terms one another,

Therefore, the number of ways is
= 210 +252+210+120+45+10+1=848 ways
I believed you have understood it.
Now try these questions ;
b How many committees of five can be formed from 8 men and 4 women if each committee is to have at least 2 women?
If you have any questions, please drop them in the comment section.
Solution of the exercises will be posted in my next post
Pictures: All pictures used in this post was created by me using math editor app.
Follow @hayzeed and visit my blog daily for more tutorial.
You can also read:
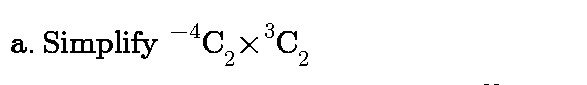
Congratulations! This post has been upvoted from the communal account, @minnowsupport, by Hayzeed from the Minnow Support Project. It's a witness project run by aggroed, ausbitbank, teamsteem, theprophet0, someguy123, neoxian, followbtcnews, and netuoso. The goal is to help Steemit grow by supporting Minnows. Please find us at the Peace, Abundance, and Liberty Network (PALnet) Discord Channel. It's a completely public and open space to all members of the Steemit community who voluntarily choose to be there.
If you would like to delegate to the Minnow Support Project you can do so by clicking on the following links: 50SP, 100SP, 250SP, 500SP, 1000SP, 5000SP.
Be sure to leave at least 50SP undelegated on your account.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit