Mathématiques : algèbre linéaire 2 - Qu'est-ce qu'une famille génératrice ? -

Récemment, je vous ai expliqué ce qu'était une famille libre ou liée dans un espace vectoriel en algèbre linéaire. Il est temps maintenant de s'intéresser aux familles génératrices. Je vais essayer de vous expliquer cela en limitant au maximum l'abstraction et les définitions trop lourdes. 

Considérons un corps K et un espace vectoriel E pour tout ce qui suit. La notation vectorielle dans cet article est abandonnée pour des raisons typographiques. 

Prenons les trois vecteurs de la base canonique dans R3 

Soient i (1,0,0) , j (0,1,0) et k (0,0,1) 

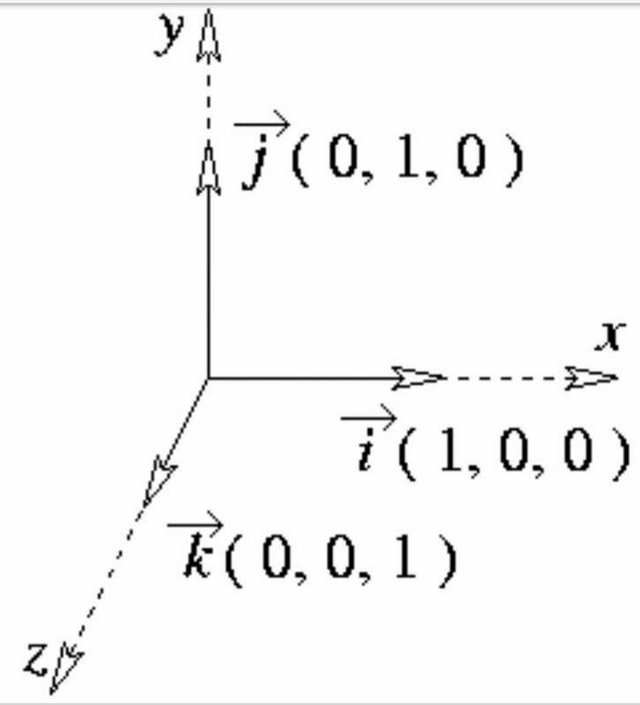
Quelle est la particularité de cette famille de vecteurs nous concernant présentement ? 

Eh bien, grâce à eux, nous pouvons construire n'importe quel point de l'espace. Prenons un vecteur arbitraire u (7, 5, 4) pour nous en convaincre : 

Nous avons u = 7i + 5j + 4k 

Plus généralement, tout vecteur V (x,y,z) peut s'écrire comme une combinaison linéaire des vecteurs de la base canonique : 

V = xi + yj + zk 

Sans le savoir, nous venons de montrer que les vecteurs de la base canonique dans R3 sont une famille génératrice. 

Une famille génératrice est donc une famille de vecteurs qui a pour faculté de construire tous les autres vecteurs, par combinaison linéaire, de l'espace vectoriel considéré. Ceci est valable pour tout espace vectoriel de dimension finie. Ainsi on peut par exemple trouver une famille génératrice dans l'espace des matrices carrées.(eh oui, une matrice est un vecteur...) 

La question est maintenant de savoir comment montrer qu'une certaine famille de vecteurs est une famille génératrice. Prenons un exemple : 

Soient i (2,-1,3) j (-1,4,-3) k (1,0,1) 

Cette famille de vecteurs est-elle une famille génératrice de R3 ? 

Il existe différentes façons de montrer cela, dont certaines assez subtiles mais je vais néanmoins vous montrer la moins élégante (car c'est la méthode de base et elle ne demande pas de compétences particulières) 

Soient a,b,c,x,y,z dans R 

Nous devons résoudre le système suivant : 

a (2,-1,3) + b(-1,4,-3) + c (1,0,1) = (x,y,z) 

Ce système s'écrit : 

2a - b + c = x 

-a + 4b = y 

3a - 3b + c = z 

La question qui vient à l'esprit est : pourquoi faut-il résoudre ce système pour montrer que la famille est génératrice (ou pas) ? 

En fait, l'idée est de montrer que pour des x,y,z arbitraires, nous pouvons toujours trouver des coefficients a,b,c pour construire notre vecteur comme combinaison linéaire des i,j,k. Si la famille n'est pas génératrice, alors le système ci-dessus est incompatible. 

Après résolution (je vous fais grâce des calculs) nous trouvons : 

 a = -2x + y + 2z
 b = (-x + y – z)/2
 c = 4.5x -1.5y -3.5z 

Nous voyons, oh magie, que peut importe nos x,y,z de départ, nous arrivons toujours à exprimer a,b,c en fonction d'eux. C'est gagné. La famille est donc génératrice et elle possède la même faculté que les vecteurs de la base canonique, à savoir construire l'intégralité de l'espace R3. 

On verra dans un prochain article à quoi servent concrètement une famille libre et une famille génératrice et notamment une propriété fondamentale de l'algèbre linéaire qui en découle directement.