
The tuba was a fairly late addition to the symphony orchestra. For the first three or four decades of the nineteenth century, composers relied on the serpent, the ophicleide, the English bass horn or the Russian bassoon to provide their brass sections with a bass or contrabass.
The serpent was a woodwind instrument, and therefore not at all satisfactory for this role. Berlioz (242) described its tone as “cold, horrible bawling.” Its lowest note—A1, three ledger lines below the bass clef—was a perfect fourth above the lowest note of the four-stringed double bass, so it did not even possess a particularly deep register.
The English bass horn was a popular variant of the serpent. It had the same range but was made of brass. The Russian bassoon was of a similar “serpentine” character, but its lowest note was D2, a perfect fourth higher than the serpent’s lowest note. Forsyth (175) describes both as “experimental or transitional types which soon lost ground when opposed by the more perfect Ophicleide.”
The bass ophicleide, which only made its appearance in 1817, had virtually the same range as the serpent, so it was no improvement on the latter as far as register was concerned. Being essentially a large keyed bugle, however, it was at least a true brass instrument. Its tone was considered superior to that of the serpent and its offspring, and it made frequent appearances in nineteenth-century scores. For example:
- Spontini, Olimpie (1819)
- Mendelssohn, A Midsummer’s Night Dream (1826, 1842—originally for English bass horn)
- Auber, La Muette de Portici (1828)
- Berlioz, Symphonie Fantastique (1830)
- Halévy, La Juive (1835)
- Bellini, I Puritani (1835)
- Meyerbeer, Les Huguenots (1836)
- Wagner, Rienzi (1842)
- Balfe, The Bohemian Girl (1843)
- Adam, Le Corsaire (1856)
- Arthur Sullivan, Overture di Ballo (1870)
- Verdi, Requiem (1874)
- Saint-Saëns, Samson et Dalila (1877)
There was nothing wrong with the ophicleide that required its replacement in the orchestra. Instead, a successful marketing campaign representing the Tuba as a more up-to-date instrument forced its replacement. (Brendan Lai-Tong)
The fact that leading composers continued to score for the ophicleide well into the 1870s lends some support to this assertion.
Although there did exist a contrabass serpent and a contrabass ophicleide, which extended the range of these instruments by an octave, they were much too rare to become established members of the orchestra:
Mendelssohn clearly wished to strengthen his orchestra’s lower register, yet only one contrabass serpent (c. 1840) and one contrabass ophicleide (c. 1834) are known to have existed, both of them in England. (Bevan 147)
It was to fill this hole in the lower register of the brass section that the Prussian bandmaster Wilhelm Friedrich Wieprecht and the Berlin instrument maker Johann Gottfried Moritz created the world’s first bass tuba:
For ten years now I have been working with military bands, and have felt, I suppose, most sorely the need of a true contrabass [brass]wind instrument ... this would surely indicate how important and advantageous for music is the invention of the chromatic Bass-Tuba, which can descend one octave lower than the serpent and English bass horn, and six notes lower than the ophicleide, while yet retaining the high notes of these three said instruments. (from Wieprecht’s patent application, quoted in Bevan 147-148)
The Bass Tuba in F was born on 12 September 1835, the day on which Wieprecht and Moritz’s Prussian Patent Number 9121 was granted. Although this was not the world’s first tuba—the Bohemian instrument maker Václav František Červený had invented a contrabass tuba in 1834 (Phillips & Winkle 5)—it was the first bass tuba. Johann Moritz died in 1835, but he was succeeded by his son Carl Wilhelm, whose firm, C W Moritz of Berlin, constructed 84 bass tubas between then and 1845 (Holcomb).
The Bass Tuba of Wieprecht and Moritz
The fundamental tone of the original bass tuba was F1, or 12' F, which lies one octave below the bass clef. The harmonic series of the instrument was as follows (with black note-heads for those harmonics that were significantly out of tune):
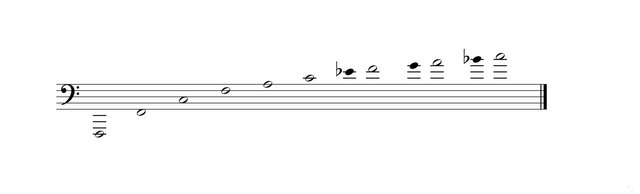
This was a whole-tube instrument: that is to say, the fundamental tone could be played. In order to fill in the notes between the harmonics, the instrument was fitted with five valves of the Berliner Pumpen type—invented by Wieprecht and Moritz in 1833—that were the forerunners of the modern piston valve. By engaging one of the pistons that operated these valves, the airflow through the instrument was diverted through an extra piece of tubing. This had the effect of increasing the overall length of the tuba, and thus lowering the pitch of whatever harmonic was being played at the time. Prior to the invention of valves, brass instruments were limited to the “open” notes of the harmonic series.
The introduction of valves, however, was a mixed blessing, as it brought with it intonational problems—problems which nineteenth-century instrument makers were hard pressed to solve.
The main problem was that while the extra piece of tubing introduced by engaging a particular valve was of the correct length when only that valve was engaged, it was too short when that valve was engaged at the same time as another valve. The result was that when valves were used in combination, the resulting tones were too sharp. Sometimes a skillful player could “bend” the note back into tune using his lips or embouchure. This technique is called lipping, and can adversely affect tone quality. On the horn, intonation could be corrected quite easily by inserting the hand into the bell, but this practice was not usually available to trumpeters or tubists. Nevertheless, there is much evidence that brasswind players often used valves in combination, even when they were not designed to be so used.
Adolphe Sax’s solution to the problem was a system of six independent valves. These valves were designed to be used one at a time, and never in combination. They actually diverted the airflow through shorter lengths of tubing, so that they raised, rather than lowered, the open notes by 1, 2, 3, 4, 5 and 6 semitones. This was sufficient to bridge the gap—a perfect fifth—between the second and third harmonics. On a whole-tube instrument like the tuba, however, this was not enough to bridge the gap—one octave—between the fundamental and the second harmonic. Sax, however, was mainly interested in applying his system to half-tube instruments, whose fundamentals were not available. Sax’s independent system never really caught on, and it was eventually superseded by the introduction of compensatory valves.
Another solution to the problem of intonation, compensatory valves were designed to be used in combination without the intonational problems that arose when independent valves were used in combination. True compensatory valves first appeared around 1858, but it was not until 1878, when D J Blaikley patented his system of automatic compensation, that they were routinely applied to tubas. (Encyclopaedia Britannica 1911, “Valves”)
A Few Words About Valves and Intonation
Imagine we have a very simple brasswind instrument consisting of nothing more than a cylindrical pipe open at both ends. The column of air inside this pipe can be made to vibrate in different modes, each mode corresponding to one of the notes of the harmonic series. Let us assume that the pipe is precisely 3540 mm long. Herbert & Wallace (149) give this as the overall length of the Moritz bass tuba in 12' F. Let us further assume that the fundamental note of this pipe’s harmonic series is F1, or 12' F. Finally, let us assume that we wish to alter the length of this instrument so that we can play all the notes of the tempered scale between this fundamental and the second harmonic, F2, one octave higher.
The ratio between the fundamental pitches of two pipes identical in all respects but their length is related to the ratio of their lengths. The longer such a simple pipe is, the lower its fundamental pitch. In our system of tempered intervals, the ratio for two pipes whose pitches are one semitone apart is:
In other words, if we wish to lower the pitch of our imaginary instrument, we must increase its length by a factor of about 1.056463. To the nearest millimetre, this means increasing the base length of 3540 mm to a new length of 3750 mm. This is essentially what the valves do on real brasswind instruments.
To lower the pitch by another semitone we must lengthen this new piece of tubing by a factor of 1.056463, or—which is mathematically the same thing—lengthen the original pipe by a factor of 22/12 . Either way we get a new length of 3974 mm. To lower the pitch of the original pipe by three semitones, we must increase its length by a factor of 23/12. And so on.
Now, in order to be able to play all eleven notes between the fundamental note (F1) and the second harmonic (F2), we need to be able to lengthen the original pipe eleven times. This is tantamount to providing our hypothetical instrument with eleven independent valves. The following table gives the lengths of additional tubing required for each of these notes, and the resulting overall length of the instrument.
| Note | Valve Length/mm | Pipe Length/mm |
|---|---|---|
| F2 | 0 | 3540 |
| E2 | 210 | 3750 |
| E2-flat | 434 | 3974 |
| D2 | 670 | 4210 |
| D2-flat | 920 | 4460 |
| C2 | 1185 | 4725 |
| B1 | 1466 | 5006 |
| B1-flat | 1764 | 5304 |
| A1 | 2079 | 5619 |
| A1-flat | 2414 | 5954 |
| G1 | 2768 | 6308 |
| G1-flat | 3143 | 6683 |
| F1 | 0 | 3540 |
F1 and F2 are the first two harmonics, so they are produced using the original 3540 mm pipe without the need to engage any valves. In order to sound any one of the eleven pitches between these two notes, the player must blow the second harmonic, F2, while engaging the appropriate valve. Engaging a valve—for example, the 1185 mm valve 5-semitone valve, in order to play C2—is essentially the same as changing the original instrument from a tuba in 12' F to one in 16' C, and playing the second harmonic of this new instrument.
Note that if a player tried to lower the pitch of an open note by three semitones by using the 210 mm 1-semitone valve and the 434 mm 2-semitone valve in combination, the overall length of the tube would only be increased to 4184 mm, whereas the required length for this note is 4210 mm. The ratio between these two is 1.006134. This is about 10.7 cents, a difference in pitch which is just about audible to most people. (A semitone in our equally-tempered system of intonation is exactly 100 cents.)
If the first three valves were used in combination to lower the pitch by a total of six semitones, the resultant length of the tube would be 4854 mm, whereas the correct length is 5006 mm, a difference in pitch of more than 53 cents, or about a quarter tone. As more and more independent valves are used in combination, the intonational problems become greater and greater.
This analysis has of necessity been simplified. Actual brasswind instruments do not consist of open-ended cylindrical pipes. Even if most of the tubing is of cylindrical bore, there will always be a mouthpiece at one end, a bell at the other end, and a section of tubing—the flare—adjoining the bell where the bore profile is not cylindrical. The player’s mouth necessarily closes off one end of the tube. Now, closed pipes do not resonate like open pipes: their natural modes of vibration only include the odd-numbered members of the harmonic series. To generate a complete harmonic series, the instrument’s natural harmonics are forced into line by the acoustical effects of the bell, flare and mouthpiece. The mouthpiece effect forces the higher harmonics down in pitch, while the corresponding bell and flare effect forces all harmonics up and also helps to generate the instrument’s pedal tone (ie the fundamental of the resulting harmonic series). A brass instrument’s harmonic series, then, is not its natural series, but an artificial construct.
The extra sections of tubing that are added to the instrument when a valve is engaged are usually of cylindrical bore. This has two different effects. Firstly, it increases the overall length of the tube, which lowers the pitch. Secondly, it increases the proportion of the tube that is of cylindrical bore. This too can affect both the pitch and the timbre of the instrument in a manner that is not easy to model. So the acoustical principles of these instruments are much more complicated than my simple figures imply:
This brings us to the problem of using valves in combination: if a valve adds the correct amount of tubing to lower the instrument by x semitones, it will not add enough tubing to lower the instrument by x semitones if another valve is in use at the same time. The calculations are more complex than the simple proportions often presented in the literature, since valves increase the amount of cylindrical tubing in the windway and affect the bore profile as well as the air-column length. (Myers 128)
The Valves on the Moritz Bass Tuba
So much for the acoustics of brasswind instruments. What about the original bass tuba that Johann Moritz constructed for Wilhelm Wieprecht?

This instrument was pitched in 12' F, was about 3540 mm long, and was fitted with five valves. It seems that these valves comprised an early form of compensatory system. The Vienna Symphonic Library has this to say about the early history of the modern tuba:
The modern tuba is the result of numerous attempts to make a bass instrument that was capable not only of producing a sound that carried well but also enabled satisfactory intonation. In 1835 Johann Moritz and the trombonist Wilhelm Wieprecht (1802–1872) were granted a Prussian patent in Berlin for the bass tuba. This instrument was made of brass, keyed in F and had five piston valves (“Berlin valves”).
The 1st and 2nd valves lowered the fundamental note by a whole tone and a semitone respectively in relation to the key of F; the 3rd valve lowered the fundamental pitch by a fourth, from F to C. The 4th and 5th valves lowered the pitch from C by a whole tone and a semitone (wide half step, wide whole step) respectively. The relatively narrow bore meant that the bass notes were not particularly loud or powerful.
This means that the semitone by which Valve 5 (V5) lowered the pitch was somewhat larger than the semitone by which V2 lowered it. Similarly, the whole tones by which V1 and V4 lowered the pitch were also unequal in size. The only practical reason for providing and instrument with two semitone valves and two whole-tone valves is to correct the intonational problems that would arise when valves were used in combination. V4 and V5 were designed to work in combination with V3.
On a popular online forum devoted to all things tuba—TubeNet—I came across a Swedish tuba in F with the same tuning as that described by the Vienna Symphonic Library. Although manufactured in 1890, it closely resembles the Moritz original of 1835. The valves, two for the left hand (L1 and L2) and three for the right hand (R1, R2 and R3) lower the pitch by the following amounts:
- L1 (V1): whole step
- L2 (V2): semitone
- R1 (V4)): long whole step
- R2 (V5): long semitone
- R3 (V3): fourth (5 semitones)
Petter Utne Borrehaug, the Norwegian instrument maker who restored this tuba, summed this up as follows:
Basically a double F & C tuba with each side having 2 valves and the R3 acting as the shift valve.
This is reminiscent of the following description of the original bass tuba of Wieprecht and Moritz:
Along with the instrument maker Johann Moritz, Wieprecht constructed an instrument pitched in F and C... with five valves (a common number even today, the additional valves controlling the tubing that provides the lower notes). (Bevan 148)
A similar account can be found on an Austrian online lexicon of musical terms (Oesterreichisches Musiklexikon ONLINE) under the headword Tuba. This article also adds that Wieprecht’s later six-valve systems were developed in an attempt to overcome the intonational weaknesses inherent in his five-valve system.
Let us see how such a five-valve system might have worked in practice. We will once again use our imaginary instrument, comprising an open cylindrical pipe 3540 mm long.
| Valve | Valve Length/mm | Pipe Length/mm |
|---|---|---|
| V1 | 434 | 3974 |
| V2 | 210 | 3750 |
| V3 | 1185 | 4725 |
| V4 | 579 | 4119 |
| V5 | 281 | 3821 |
Could these valves be used in various combinations to fill in the octave between the second harmonic and the fundamental? Let us see:
| Note | Valve(s) | Total Valve Length/mm | Total Pipe Length/mm | Correct Length/mm | Discrepancy/cents |
|---|---|---|---|---|---|
| F2 | - | 0 | 3540 | 3540 | 0 |
| E2 | V2 | 210 | 3750 | 3750 | 0 |
| E2-flat | V1 | 434 | 3974 | 3974 | 0 |
| D2 | V1+V2 | 644 | 4184 | 4210 | 11 |
| D2-flat | V1+V2+V5 | 925 | 4465 | 4460 | -2 |
| C2 | V3 | 1185 | 4725 | 4725 | 0 |
| B1 | V3+V5 | 1466 | 5006 | 5006 | 0 |
| B1-flat | V3+V4 | 1764 | 5304 | 5304 | 0 |
| A1 | V1+V2+V3+V5 | 2110 | 5650 | 5619 | -10 |
| A1-flat | V1+V2+V3+V4 | 2408 | 5948 | 5954 | 2 |
| G1 | V1+V2+V3+V4+V5 | 2689 | 6229 | 6308 | 22 |
| G1-flat | V1+V2+V3+V4+V5 | 2689 | 6229 | 6683 | 122 |
| F1 | - | 0 | 3540 | 3540 | 0 |
So whilst using these valves in combination does not solve all the intonational problems, it comes quite close. Of the eleven chromatic notes between F1 and F2:
- Five are in tune
- Two are only two cents out of tune, which is too small an interval to be audible
- Three are 10-22 cents out of tune, which is audible but can be corrected by lipping
- One is more than a semitone out of tune, and probably unusable
The instrument, therefore, was almost chromatic and in tune from the fundamental. Only one note in the bottom octave was not available. I am assuming that a good player could bend an out-of-tune note into tune if the discrepancy was no more than about 65 cents. The ease with which, and the extent to which, players could lip wayward notes into tune on early brass instruments was examined by L Norman et al (2010):
There is much debate concerning the extent to which players attempted to ‘correct’ the tuning of these wayward notes, either through pitch bending due to embouchure manipulation, using hand technique, or not at all. By manipulating the embouchure, a skilled player can, to some extent at least, bend certain notes to more closely match those of a tempered scale. The ease with which this can be achieved depends on a number of factors, including the bore profile, which largely determines the frequencies and bandwidths of the resonances. (Norman et al, Abstract)
Players were asked to bend various harmonics upwards and downwards as much as they could on eighteenth-century horns:
Figure 5 below shows an image of the varying spectral content, measured in the mouthpiece, of the 4th resonance of a horn in D as it lipped upwards ... As time increases, so too does the pitch. In this example the pitch varies by approximately half a semitone, rising from 148 Hz to 153 Hz ... At the point of maximum upward bend, where the player feels that the note is on the edge of jumping to the next highest resonance ... the fundamental frequency has risen from 136 Hz to approximately 141 Hz. (Norman et al 3)
153:148 is about 58 cents, which is indeed “approximately half a semitone”, while 141:136 is about 63 cents.
Humans can distinguish a difference in pitch of about 5-6 cents. (Loeffler 6)
Sealy Begs to Differ
But is the tuning we have just examined accurate? Further online research turned up the following passage in a Masters thesis by Byford Gayle Sealy:
The instrument was quite different from the bass tuba as we know it today. It was in the key of F and had five valves, two operated by the left hand and three, by the right hand. The valves lowered the open notes of the instrument as follows:
- 1st valve—one tone.
- 2nd valve—one semitone.
- 3rd valve—two tones when combined with the first valve.
- 4th valve—one and a half tones when combined with the first valve.
- 5th valve—a perfect fifth.
The source Sealy cites for this information is page 305 of Musical Wind Instruments by Adam Carse, but I cannot find any such statement anywhere in this work. For the sake of argument, however, let us suppose that Sealy’s information is accurate. How will that work out on our 3540 mm pipe?
| Valve | Valve Length/mm | Pipe Length/mm |
|---|---|---|
| V1 | 434 | 3974 |
| V2 | 210 | 3750 |
| V3 | 486 | 4026 |
| V4 | 236 | 3776 |
| V5 | 1764 | 5304 |
Could these valves be used in combination to fill in the octave between the second harmonic and the fundamental? Let us see:
| Note | Valve(s) | Total Valve Length/mm | Total Pipe Length/mm | Correct Length/mm | Discrepancy/cents |
|---|---|---|---|---|---|
| F2 | - | 0 | 3540 | 3540 | 0 |
| E2 | V2 | 210 | 3750 | 3750 | 0 |
| E2-flat | V1 | 434 | 3974 | 3974 | 0 |
| D2 | V1+V4 | 670 | 4210 | 4210 | 0 |
| D2-flat | V1+V3 | 920 | 4460 | 4460 | 0 |
| C2 | V1+V3+V4 | 1156 | 4696 | 4725 | 11 |
| B1 | V1+V2+V3+V4 | 1336 | 4906 | 5006 | 35 |
| B1-flat | V5 | 1764 | 5304 | 5304 | 0 |
| A1 | V4+V5 | 2000 | 5540 | 5619 | 25 |
| A1-flat | V1+V2+V5 | 2408 | 5948 | 5954 | 2 |
| G1 | V2+V3+V4+V5 | 2696 | 6236 | 6308 | 20 |
| G1-flat | V1+V2+V3+V4+V5 | 3130 | 6670 | 6683 | 3 |
| F1 | - | 0 | 3540 | 3540 | 0 |
Comparing these results with those for the tuning given in the previous section, we see once again that we have a mixed bag. Of the eleven chromatic notes between F1 and F2:
- Five are in tune
- Two are only two or three cents out of tune, which is too small an interval to be audible
- Four are 10-35 cents out of tune, which is audible but can be corrected by lipping
- None is more than 35 cents out of tune, and so no note is unusable
The advantage of this system is the availability of G1-flat, one semitone above the fundamental. This note is probably not available in the other system. Otherwise there is not much to choose between the two: both have seven of the eleven chromatic tones in tune. The availability of the G1-flat, however, predisposes me to lend some credence to Sealy’s tuning, even if his source is a mystery.
Baines Begs to Differ
As I mentioned above, Sealy cited Adam Carse as his authority for this tuning. It is possible, however, that he has simply mixed up his sources, because I did find the following in Brass Instruments: Their History and Development by Anthony Baines:
Originally the 1st and 2nd valves, for the left hand, lowered by a tone and by a semitone. The three for the right hand provided: a large tone, to make an exact two tones with the 1st valve; large semitone, to make an exact tone-and-a-half with the 1st valve; and a perfect fourth. (Baines 250)
This agrees with Sealy except for the size of the fifth valve: did this lower the pitch by a perfect fifth (Sealy) or by a prefect fourth (Baines)? Was this Sealy’s source, and did he simply misread it?
Let us now examine this third tuning. Remember, the only difference between this system and the one described by Sealy is the shorter length of the fifth valve:
| Valve | Valve Length/mm | Pipe Length/mm |
|---|---|---|
| V1 | 434 | 3974 |
| V2 | 210 | 3750 |
| V3 | 486 | 4026 |
| V4 | 236 | 3776 |
| V5 | 1185 | 4725 |
| Note | Valve(s) | Total Valve Length/mm | Total Pipe Length/mm | Correct Length/mm | Discrepancy/cents |
|---|---|---|---|---|---|
| F2 | - | 0 | 3540 | 3540 | 0 |
| E2 | V2 | 210 | 3750 | 3750 | 0 |
| E2-flat | V1 | 434 | 3974 | 3974 | 0 |
| D2 | V1+V4 | 670 | 4210 | 4210 | 0 |
| D2-flat | V1+V3 | 920 | 4460 | 4460 | 0 |
| C2 | V5 | 1185 | 4725 | 4725 | 0 |
| B1 | V4+V5 | 1421 | 4961 | 5006 | 16 |
| B1-flat | V1+V2+V5 | 1829 | 5369 | 5304 | -21 |
| A1 | V1+V2+V4+V5 | 2065 | 5605 | 5619 | 4 |
| A1-flat | V1+V3+V4+V5 | 2341 | 5881 | 5954 | 21 |
| G1 | V1+V2+V3+V4+V5 | 2551 | 6091 | 6308 | 61 |
| G1-flat | V1+V2+V3+V4+V5 | 2551 | 6091 | 6683 | 161 |
| F1 | - | 0 | 3540 | 3540 | 0 |
Comparing these results with those for the other tunings, we can see at once that they are somewhat inferior. Of the eleven chromatic notes between F1 and F2:
- Five are in tune
- One is only 4 cents out of tune, which is too small an interval to be audible
- Three are 16-21 cents out of tune, which is audible but can be corrected by lipping
- One is 61 cents out of tune, which is easily audible and on the limits what can be corrected by lipping
- One is 161 cents out of tune, and probably unusable
After describing the tuning of the valves, Baines continues:
Thus for D flat, 1st and 3rd; C, 5th alone; from this down to A´ flat by adding 3rd and 4th valves; and adding to these 1st and 2nd to reach low F´ sharp above the fundamental as best one could. (Baines 250)
Using V1 and V3 in combination to reach D2-flat checks out, as does the use of V5 to reach C2. But combining V3, V4 and V5 to reach A1-flat makes no sense to me: the resulting pitch is more than a semitone too sharp. A much better fit is achieved by combining V1, V2, V4 and V5—less than an eighth of a tone too sharp. Similarly, the combination of all five valves produces a pitch that is 61 cents sharper than G1 and 161 cents too sharp for F1-sharp. To produce the latter note, it would make more sense to try and bend the fundamental up than to engage all five valves and bend the resulting G1 pitch down—though both attempts would be futile, as the discrepancies are too great to be lipped.
Who Do We Believe?
Comparing all three systems, it seems that Sealy’s tuning is the best after all, even if his source is obscure. Summing up the accumulated discrepancies in cents, we get:
- Vienna Symphonic Library: 169 cents
- Sealy: 96 cents
- Baines: 284 cents
Most of these discrepancies, however, involve the bottom few notes. If we discount the low G1-flat, the “scores” for the three systems are quite different:
- Vienna Symphonic Library: 47 cents
- Sealy: 93 cents
- Baines: 123 cents
Now it is the Vienna Symphonic Library’s tuning that wins.
According to yet another source—the online Encyclopaedia Britannica, “The history of Western wind instruments: The Romantic period”—the instrument did not even go as low as G1:
The invention of the bass tuba by a German instrument builder named Johann Gottfried Moritz in 1835 provided a more reliable and more even brass bass. Tuned in F or E-flat, it had a range that was, respectively, [B1-flat to E4-flat] ...
If this were true, there would be very little to choose between the three tunings we have examined. But I don’t think this is true. Wagner, for instance, takes his bass tuba down to A1-flat in A Faust Overture (1839-40), an early work singled out for special mention by Anthony Baines in connection with the Moritz instrument:
Moritz’s tuba won much admiration and must surely have been envisaged by Wagner in the opening of the Faust Overture, which no contemporary bombardon could have managed. (Baines 250)
So, who do we believe? Although the first system is not as good as Sealy’s, I am inclined to think that it was the one Wieprecht designed for his original bass tuba. I still don’t know what source Sealy used for his tuning, but I have found several independent sources to support the other tuning. And if Wieprecht’s original five-valve system had been as good as Sealy’s, would he have experimented with six-valve systems in an attempt to correct its intonational weaknesses?
Surviving Specimens of the Moritz Bass Tuba
Several specimens of Moritz’s pioneering bass tuba in F are extant, so the possibility of clearing up these inconsistencies is tantalizingly within reach. A particularly fine specimen is on display at the Musical Instrument Museum in Brussels. Surely it would not be asking too much to have a professional tuba player blow into it in order to determine precisely what pitches it can sound! Violins made by Stradivarius are still in active use, so there should be little danger of damaging the instrument.
The renowned Hungarian tubist Roland Szentpáli has an early Moritz tuba in E-flat. In this YouTube video he performs a piece by Marco Bordogni’s: Thirty-Six Vocalises in the Modern Style, Number 31 in E-flat, Andante sostenuto e Allegro. The performance took place on 16 February 2014 in the Musikinstrumenten-Museum, Berlin. the pianist is Krisztina Fejes:
Prussian Patent #9121
Wieprecht’s patent documents are an important primary source for information about the instrument. I believe they are archived at the Prussian Privy State Archives in Berlin. I have been unable to track down an online version, so all I have to go on are a handful of sporadic quotations that have appeared in the literature.
But even here we find statements that are difficult to reconcile with reality:

... this would surely indicate how important and advantageous for music is the invention of the chromatic Bass -Tuba, which can descend one octave lower than the serpent and English bass horn, and six notes lower than the ophicleide, while yet retaining the high notes of these three said instruments. (from Wieprecht’s patent application, quoted in Bevan 148)
Both the serpent and the bass ophicleide could descend to A1 (three ledger lines below the bass clef). Using the five valves in combination, the fundamental of the Moritz bass tuba, F1, could be lowered—theoretically, at least—to G0 or G0-flat, so perhaps Wieprecht is justified in his claim. But Walter Piston (285) advises the composer not to write lower than D1 (a minor third below the fundamental) when scoring for the modern bass tuba in 12' F. Forsyth is even more damning. After describing how the valves can be used to fill in the octave between the second harmonic and the fundamental, he notes:
He [ie the player of the modern bass tuba in 12' F] can then take off all his valves, and, with a clear bore, produce his fundamental note. Below this there are some few notes which can be got by means of a very loose lip, but their quality is, as a rule, miserable and their intonation unsatisfactory. Still they are played. (Forsyth 156)
Upper Range

In this article I have concentrated on the lower range of the Moritz bass tuba in F, as this is the register in which most of the uncertainties lie. Before signing off, however, it would not be amiss to say a few words about the upper range of the instrument.
In his patent application Wieprecht boasted that his bass tuba could match the serpent and ophicleide in this range. Forsyth (174, 287) gives B4-flat (middle line of the treble clef) as the uppermost note on both the ophicleide and the serpent. Berlioz (233) extends the ophicleide’s range a whole tone higher, to C5. The latter is the twelfth harmonic of the Moritz tuba. Piston recommends that the modern bass tuba in F be taken no further north than the “eighth partial” (ie the ninth harmonic, or G4), but it was probably easier to play higher than this on Moritz’s tuba, which had a narrower bore than today’s instruments.
In his overture to Die Meistersinger von Nürnberg, which was composed in 1862, Wagner takes the bass tuba no higher than E4. In Act I, Scene III, it reaches F4, the eighth harmonic—and that is all he wrote.
And that is all I have written.
References
- Anthony Baines, Brass Instruments: Their History and Development, Dover Publications Inc, New York (1976)
- Hector Berlioz, Hugh Macdonald (translator), Berlioz’s Orchestration Treatise: A Translation and Commentary, Cambridge University Press, Cambridge (2002)
- Clifford Bevan, “The Low Brass”, in Herbert & Wallace.
- Marco Bordogni, Vocalizzi secondo il gusto moderno (36 Vocalises in the Modern Style), Number 31 in E-flat
- Adam Carse, Musical Wind Instruments
- Trevor Herbert, John Wallace (editors), The Cambridge Companion to Brass Instruments, Cambridge University Press, Cambridge (1997)
- Philip Holcomb, Tuba von 1850, Accessed 15 November 2016. See also Holcomb’s website at Rugs-n-Relics
- Brendan Lai-Tong, Ten Tuba Facts, Take Note, Accessed 16 November 2016
- Beatus Dominik Loeffler, Instrument Timbres and Pitch Estimation in Polyphonic Music, Master Thesis, School of Electrical and Computer Engineering, Georgia Institute of Technology, May 2006
- Arnold Myers, “Design, Technology and Manufacture Since 1800”, in Trevor Herbert, John Wallace (editors), The Cambridge Companion to Brass Instruments, Cambridge University Press, Cambridge (1997)
- L Norman, J P Chick, S Logie, D M Campbell, Pitch Bending on Early Brass Instruments, Proceedings of 20th International Symposium on Music Acoustics (Associated Meeting of the International Congress on Acoustics), 25-31 August 2010, Sydney and Katoomba, Australia
- Harvey Phillips & William Winkle, The Art of Tuba and Euphonium, Summy-Birchard Inc, Los Angeles (1992)
- Vienna Symphonic Library
Image Credits
- Bass Tuba in F by J G Moritz: The original F-Tuba of J G Moritz and W Wieprecht. Serial Number 64 (September 1835), Public Domain
- Bass Tuba in F1 by Carl Wilhelm Moritz, Berlin (between 1838 and 1840) : © 2016 Staatliches Institut für Musikforschung
- Wilhelm Wieprecht : Wood Engraving (1872), Public Domain
- Bass Tuba in F by C W Moritz: Horn-U-Copia
Video Credits
- Szentpáli Plays His Moritz E-flat Tuba: Roland Szentpáli, Standard YouTube License