6 math operations are solved in an order, known as order of operations

As you already know that addition, subtraction, multiplication and division are the four basic operations in mathematics. In elementary grades (from grade one to five) students learn these operations one by one. Once the students move to grade six, they start to see all of these operations combined in the math problems. The problems become more complex when brackets (parenthesis) and exponents are introduced in grade six.
Now with four basic operations, the students have to deal with brackets and exponents too. At this stage, students need encouragement and appreciation for their efforts and motivation. Because in lower grades, some topics, such as leaning times tables or prime factorization were not less challenging. If the students have learned those, learning all operations together is not that hard either. The need is just for self-confidence, motivation and hard work.
Approaching these complex problems properly and systematically makes the students’ work lot easier.
The order of operations is a “must know” for grade six or higher grades students to make the complex problems (having the mix of all the four operations, brackets and exponents) easy.
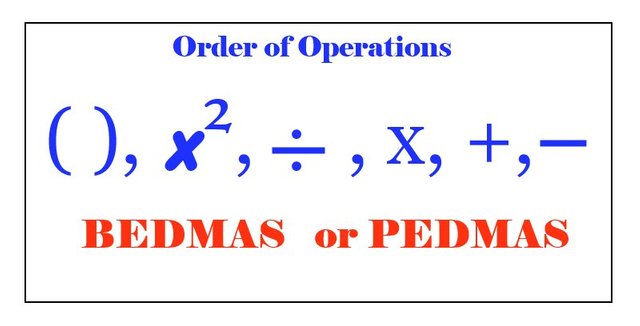
To memorize the order of operations, the word “BEDMAS” OR "PEDMAS" can be memorized. Both words give you the same result, the difference is the words "P" and "B". P Stands for Parentheses whereas B stands for Brackets; both means one and the same thing. This word is a code word for all the operations and it is decoded below:
B means Brackets (P means Parentheses): If there are brackets (parentheses) present in the problem, always get rid of them, first of all.
E means Exponents: After solving the brackets, look for exponents in the question and solve them. By the way, exponents are the powers raised to the numbers.
D means Divide: Once the brackets and exponents (powers) are gone, look for divide signs and solve the parts of the question which have divide signs.
M means Multiplication: After solving for divide signs, solve the parts of the problem having multiply signs.
A means Add: Once you have solved for brackets, exponents, divide and multiply, the only signs you see are plus and minus. Add the numbers together having the plus signs at their front.
S means Subtract: Finally you will end up with all the negative numbers and one positive number you obtained from the previous step. Subtract the numbers to get the final answer.
Remember that, always start working on the problems from left to right. More problems you practice on order of operations, easier the process will become for you. Also you learn s lot of the new tricks by practicing more problems.
Preview: Before we start the order of operations, I like to review the rules involving brackets (parenthesis).
Following are the basic methods to solve the open brackets;
- (3+7) = 10
- 2(3+7) = 2(10) = 20
Remember that if there is no sign between a bracket and a number; then multiply (times) the numbers outside and inside the bracket.
- -3(7- 4) = -3(3) = - 9 -3(-7+4) = -3(-3) = 9
If you have problems understanding the above examples; please read the previous presentations on integers. That will help you to understand all operations on integers and above examples.
Basic order of operations: At very basic (grade 5) levels, students need to understand how to solve problems involving two operations at a time. Two operations can be any combinations of four basic operations, such as addition and subtraction or addition and multiplication.
Problems involving addition and subtraction: If you see only positive and negative signs in the problem, I highly recommend that add all the positive numbers to get one positive number. Same way add all the negative numbers to get one negative number. Now you have two numbers with opposite signs, subtract them and write the bigger number sign in front of the answer and you are done.
Let’s do the following example to understand the above concepts.
9 – 11 – 5 + 7 – 20 + 17
We have three positive numbers; 9, 7 and 17. Add these numbers together to get 33. Also, there are three negative numbers; - 11, -5 and – 20. Add these numbers together to get – 36.
This way we have converted six numbers into two numbers with opposite signs. You already know that two numbers with opposite signs are subtracted, so subtract 33 from 36 to get 3 and it will take the negative sign which is the bigger number’s sign. The given problem turns to be as given below;
- 9 -11 – 5 + 7 – 20 +17
- = 33 – 36
- = -3
Problems involving addition and multiplication or subtraction and multiplication or all three. If there is multiplication symbol involves together with positive or negative signs in the given question. Always solve the multiplication before addition and subtraction. Consider the following example to understand this.
- 2 + 3 x 5
Never ever start adding 2 and 3 in this problem.
Multiplication has the priority over add and subtract, therefore multiple 3 and 5 to get 15 first, then add 2 and 15 to get 17 as the answer. The solution is shown below;
- 2 + 3 x 5
- = 2 + 15
- = 17
Let’s do the following example problems to clear the above concepts further.
- – 6 + 4 x 5
- 12 – 3 x 7 – 1
- 9 – 2 x 16 + 50 – 33
- – 21 – 20 x (- 3) + 5 x 7
- 8 x 2 x 3 + 12(-2) - 3(-6)
Solutions:
1) There are three signs involving in this problem, the negative, positive and multiply (times). Multiplication has the priority over addition and subtraction. Therefore, do 4 times 5 to get 20. Now, you have two numbers – 6 and 20. As these numbers have opposite signs, subtract them to get 14 and number 14 is positive because bigger number 20 is positive. Mathematically, the problem is solved as below;
- - 6 + 4 x 5
- = - 6 + 20
- = 14
2)As multiplication has the priority over add and subtract, solve all the multiplication signs first of all. In the given problem – 3 is getting multiplied with 7. Solve – 3 x 7 to get -21. After this we get three numbers in the problem, 12, - 21 and -1. Add both the negative numbers -21 and -1 to get -22. Now 12 and -22 have the opposite signs, subtract them to get – 10 as your answer.
Mathematically;
- 12 – 3 x 7 – 1
- = 12 – 21 -1
- = 12 – 22
- = -10
3) In first step, solve – 2 x 16 to get – 32. Now there are four numbers in the question, 9, - 32, 50, -33. Add both the positive numbers 9 and 50 to get 59 and both negative numbers – 32 and – 33 to get – 65. So, 59 and -65, with opposite signs, need to be subtracted to get -6 as answer to the problem.
Mathematically;
- 9 – 2 x 16 + 50 – 33
- = 9 – 32 + 50 – 33
- = 59 – 65
- = -6
4) In this problem, there are two multiply signs. Solve both of multiplications first, do – 20 x (- 3) to get +60 (Note that,60 is not front of the problem but in the middle, so we have to include the plus sign with 60). Also do, + 5 x 7 to get +35 (as 35 is not in the beginning of the problem, so use the plus sign with 35) Now we end up with three numbers, - 21, +60 and + 35. Add both the positive numbers 60 and 35 to get +95. Next, – 21 and +95 have opposite signs, therefore subtract them to get 74. Notice here, the answer is +74 but we need not to show the plus sign with our final answer. Always write the plus sign in front of the number which is not at the starting of the problem as we did in case of 60 and 35 above. Mathematically;
- – 21 – 20 x (- 3) + 5 x 7
- = - 21 + 60 + 35
- = - 21 + 95
- = 74
5) Let’s do this problem step by step with explanations. 8 x 2 x 3 + 12(-2) - 3(-6) Multiply 8, 2 and 3 to get 48. Note that, there is just a bracket between +12 and – 2 and between – 3 and – 6, which means multiply as well. Therefore multiply +12 and – 2 to get – 24 and multiply – 3 and – 6 to get +18 as shown below; 8 x 2 x 3 + 12(-2) - 3(-6) = 48 – 24 +18 Now, we got three numbers with plus and minus signs only. Add the positive numbers, 48 and 18 to get 66. After this we have 66 and – 24 with opposite signs, subtract them to get 42 as the answer as shown below;
- 8 x 2 x 3 + 12(-2) - 3(-6)
- = 48 – 24 +18
- = 66 – 24
- = 42
This is very much the coverage of the basic order of operations. Keep in mind, this presentation is done considering that the reader have sound knowledge of the integers and operations with them. If you want to learn integers, read my previous presentations here, explaining integers and operations on integers.
The summary of today’s lesson is;
- If there are only positive and negative signs in the problem, add all the positive numbers to get one positive number and add all the negative numbers to get one negative number. Now subtract the two numbers you obtained above to get the answer.
- Multiplications have the priority over addition and subtraction. Never ever add or subtract the numbers until there are multiplication signs in the problem. Always multiply the numbers before you add or subtract.
Let's move further to understand more complex problems involving multiple brackets such as open brackets, square brackets and curled brackets. These brackets also have priorities among them as shown below;
- ( ) are called the open brackets (open parenthesis),
- { } are called the curled brackets, and
- [ ] are called the square brackets.
Solve these brackets in the given order, that is, solve open brackets first of all then curled brackets and finally the square brackets. Note that, some mathematicians switch between the curled brackets and square brackets. The procedure is the same, but make sure with your teacher or check your text book to find the order of last two types of brackets. Recall “BEDMAS” and get ready to learn next level of the order of operations.
Below are the examples we are going to solve:
1. 9 + 3 x (-6 + 15) – 35
2. – (- 10) – {12 – 3 (5 – 16)}
3. 25 – 20(30/6 + 2)
4. - 3 – 6[2 {- 2 – 5 (- 4 – 10 + 21/3)} + 6]
5. – (16 – 10) [5 {4 (1 + 5 x 2 – 15)}]
Let’s solve the above problems one by one.
1) 9 + 3 x (-6 + 15) – 35
Always look at signs at front of the numbers and types of brackets used in the problem. Start working on the problems from left to right and look for the operation that has the priority according to the “BEDMAS”. Always do the analysis of the problem as given below in your mind. You need not to write it.
In the given problem, 9 is positive, positive 3 is getting multiplied to the open bracket, negative 6 and positive 15 are inside the bracket and finally there is negative 35 at the end.
After analyzing the problem start your solution. Solve the open bracket first of all and get 9 for the bracket, as – 6 + 15 = 9.
So, the problem becomes as given below;
= 9 + 3 x 9 – 35
Note that, I rewrote the rest of the numbers from the first step and solve the bracket only.
Our next prey is times sign; 3 x 9 = 21 (the answer 21 is positive as 3 and 9 are both positive).
Therefore our problem shrinks to;
= 9 + 27 – 35
= 36 – 35 = 1
Again, 9 and 27 are both positive, hence I added them to get positive 36 and 36 – 35 = 1, is our answer.
On the next problems I will explain the math steps only in brief.
2) – (- 10) – {12 – 3 (5 – 16)}
= 10 – {12 – 3 (- 11)} Remember – (- 10) = 10 and 5 – 16 = - 11
= 10 – {12 + 33} Because - 3 multiplied by – 11 or – 3(- 11) = 33
= 10 – (45)
Note: 12 and 33 are both positive so I added them to get plus 45. Another thing, once you got rid of open bracket you can switch curl bracket or square bracket to open bracket. That’s why I wrote (45) instead {45}. Now next step of solution is below:
= 10 – 45
= -35
Note – ( 45) = - 45 and 10 and 45 have opposite signs, so subtract 10 from 45 to get 35 and 35 takes the bigger number sign which is negative from 45.
3) 25 – 20 (30/6 + 2)
= 25 – 20 (5 + 2) Because 30/6 = 5
= 25 – 20 (7)
= 25 – 140
= - 115
4) - 3 – 6[2 {- 2 – 5 (- 4 – 10 + 21/3)} + 6]
In this multiple bracket problem solve the open bracket first of all and in that get 21/3 = 7
= - 3 – 6 [2 {- 2 – 5 (- 4 – 10 + 7)} + 6]
= - 3 – 6 [2 {- 2 – 5 (- 7)} + 6]
I solved – 4 – 10 +7 to get – 7 in the open bracket. Further, solve – 5(- 7) to get 35, as bracket means multiply and both number have the same signs, so the answer is 35 and is positive. The problem is reduced to;
= - 3 – 6 [2 (- 2 + 35) + 6]
Again I changed { } to ( ) as soon as I solved the given open bracket. Now – 2 + 35 = 33 and problem becomes;
= - 3 – 6 [2 (33) + 6]
= - 3 – 6 [66 + 6]
= - 3 – 6 (72)
= - 3 – 432
= - 435
5) – (16 – 10) [5 {4 (1 + 5 x 2 – 15)}]
= - (6) [5 {4 (1 + 10 – 15)}]
= - 6 [5 {4 (- 4)}]
= - 6 [5 (- 16)]
= - 6 (- 80)
= 480
Do you mean PEMDAS? Writing PEMDAS as PEDMAS might confuse some people.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
You are right matt-a, it can confuse. BEDMAS or PEDMAS same thing as said on first image. B is used for Brackets or Instead P can be used for Parenthesis which makes PEDMAS instead of BEDMAS. One and the same thing.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
In the USA people are taught the order of operations as PEMDAS, where as it appears in other countries people are taught BEDMAS. Yes, same thing :-D.
It's very cool that you take the time out of your life to try to teach people the beauty of math! That is a true gift.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Thanks for your suggestions matt-a. I will keep in mind to use parentheses and brackets together in my future posts.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
I have updated the post using both BEDMAS & PEDMAS. Think it will clear out any confusion. Thanks for your suggestion matt-a.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Good ! :)
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit
Thanks a lot happyphoenix. Always encouraging.
Downvoting a post can decrease pending rewards and make it less visible. Common reasons:
Submit