Imagine an empty space, in that empty space if you place a charged particle you might just start to see the particle move, more accurately accelerate. That there is an electric field, a region where a charged particle experiences a force.
Huh? How's this useful?
An application of this electric field is X-ray machines. The field accelerates electrons to crash onto metal and hence produce X-rays. And this is just one of the uses!
Now, of course, we can't see the electric fields. So instead we draw something called an electric field line to visualize the areas under the effect of the electric field.
But how do these line work?
Uhh... let's see
The arrow shows the direction in which a unipositive charge will feel the force.
Also,
lines closer together just mean stronger field and lines far away from each other means weaker field. It basically works the same way as magnetic field lines.
Wait a minute, where do these electric fields even come from in the first place?
Well, You see it is generated around a charged object. In other words, a charged object creates a field which affects other charged objects which also has an electric field around them.
There is also something else to consider here because there are two types of charges. Positive and Negative.
From a positively charged object, the electric field lines go outward on the other hand if it is a negatively charged particle then the field lines will go inwards.

And because of that, we can create a uniform electric field by lining up two plates, one with a negative charge and one with a positive charge close together. Connecting lines from one charge to the other, this a principle called superposition. BTW these pair of plates is called a capacitor if both of then are connected to the same voltage.
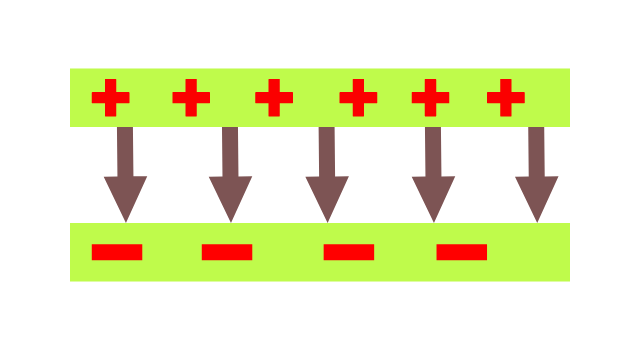
These field lines also have said properties that need to be looked at as well.
At any point, the field lines have to always be tangent at the direction of the field
What does it mean?
Picture a positive charge and a negative charge beside each other. What does the field line look like? look below
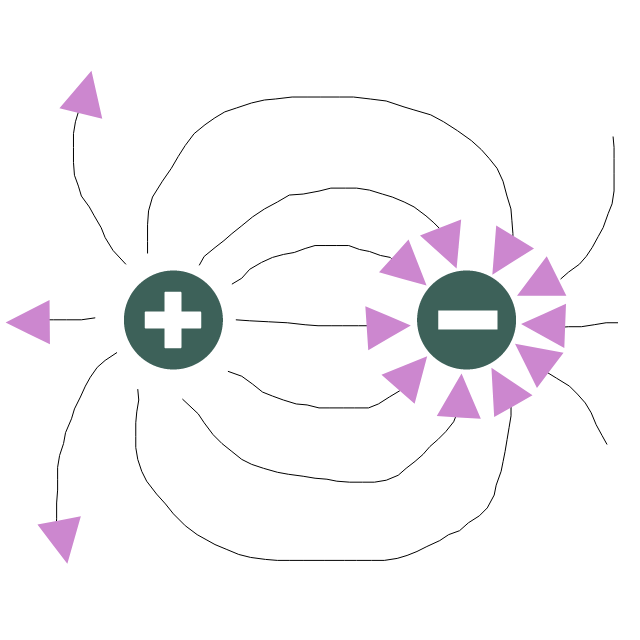
And again, This picture is what it is because of superposition. I'll talk about superposition in detail some other time.
Back to the topic at hand, say you place a unipositive charge anywhere in the field, then, of course, it'll feel a force.
But in which direction? The force is in the direction of the tangent of that field line at that point. And the opposite direction of force for a negative charge.
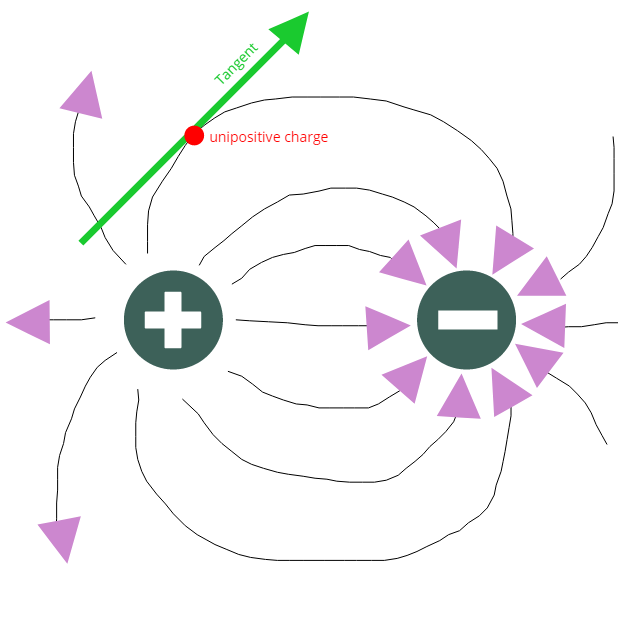
Another property
The lines always start from a positively charged to a negatively charged object. No matter what. So you might think, what if there isn't any negatively charged particle around and just positive charged particles are available. Then the lines from each particle will push each other (repulsion) while going off to infinity till it finds another negatively charged particle.
Here is again another property. As I said before the lines will push each other. Meaning the lies will never cross each other! Why? cause if they did cross, there will be a different direction of charges in the exact same location! And that's not possible!
Now, of course, there will be equations that will come into play. So, there is a force on a charge causing an acceleration.
So for the force
F=EQ (where E is something called the electric field strength, yeah the name says it all. And Q is the charge)
But how does acceleration tie into this?
everyone knows, hopefully... that F=ma (mass × acceleration)
substitute force and voila a=EQ/m
Moving onto something called Electric Potential.
Think back to the X-ray machine. There was a force on the electron, so it moved. But if it moved it must have a Kinetic Energy. Where does this energy come from? It is the cause of electric potential. And every point in the electric field gives the electron, no cut that, say any charged particle will be given a certain electric potential energy per unit of charge.
And the equation for this
V=Eₚ/Q
and so, this electric field is present between every object that has electric potential. Hence, it adds to why we could form the uniform field in the capacitor before. And here is an equation that describes this uniform electric field cause of the voltage.
E=V/d
So, as the distance between the field increases the electric field strength weakens.
This also brings something else to note. Electric field strength has two equally valid SI units cause of both the equations!
E=V/d & E=F/Q
So, NC⁻¹ & Vm⁻¹ are equally valid.
Now for a fun experiment that can enable you to visualize an electric field.
But, before that, High Voltage supplies are used in this experiment! Do at your own risk!
Take a container of clear oil and place a lot of dry small seeds or anything similar that can get charged. Add your electrodes (different shapes may shoe different lines) connected to a voltage. And see the seeds line up to represent the field lines as they get slightly charged.
Here is an image from sciencedemo.

And here is the last thing in the post I would mention.
Equipotentials
These are a line on the which goes in a perpendicular line to the field. And in that line, if you take any point and compare it with any other point on the same line, then they would have the same potential. And how close these lines are will show the electric field strength (the closer the line the more rapid change in potential there is in the direction of the field).
That's all folks