Inequality: Always, sometimes or never?

---

"Always, sometimes or never?"

"Is it always true, sometimes true or never true?"

These are questions that we should ask for everything in life, including mathematics. However, many students are accustomed to a fixed way of doing things, a fixed set of procedures to follow, a fixed type of solutions to problems. They will coast along until they encounter the following type of problem.

"What?!? I am used to seeing equations like 3 x + 1 = 7 which have one answer, and equations like x² = 9 which have 2 answers and inequalities like 2 x - 1 > 5 which has a range of answers, but not those above!?!"

Certainly so. This question is a little bit more challenging. But do not panic. Try to look for patterns. Observe that can be split up as using the difference of squares formula, with and .

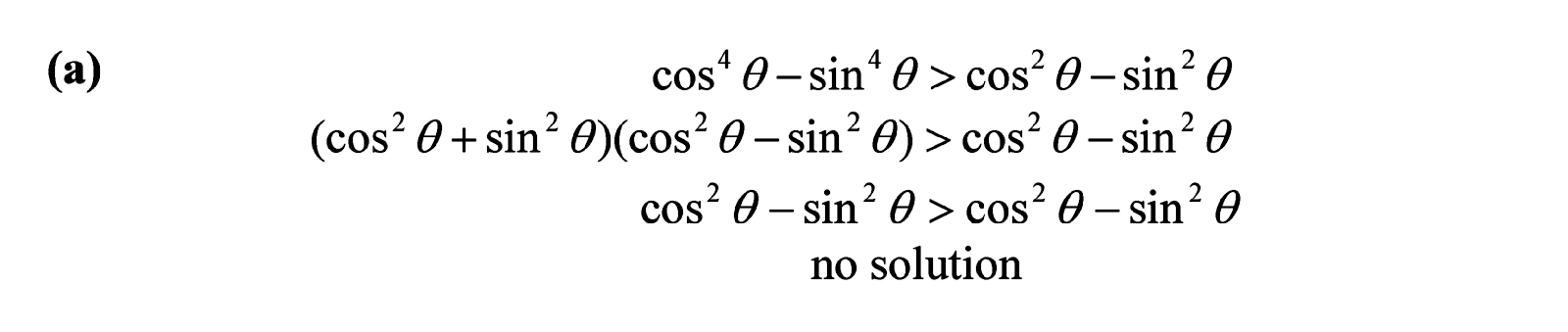
Remembering that which is an identity (so it always true), we end up with a silly statement like which is never true. So there is no solution for part (a).

For part (b) we proceed similarly.

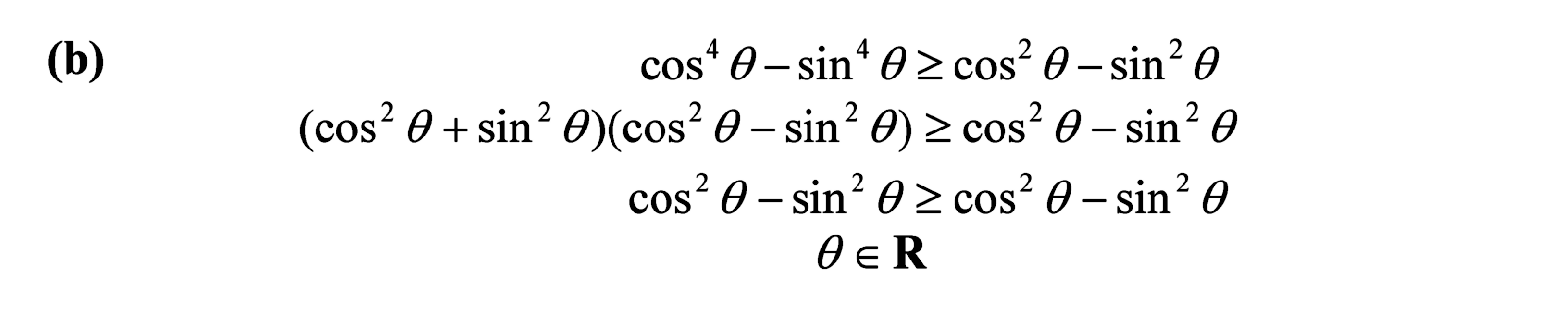
We end up with a the statement like which is always true. Everything is always greater than or equal to itself. Even though the "greater than" part is not true, the "equal" part is definitely true. So the statement is considered as true, for whatever value of theta you care to substitute in it. That means theta can be any real number. The solution set is the set of all real numbers.

Now for the clincher!

Again we proceed as in parts (a) and (b) but note that both and are equal to , which makes the Right Hand Side zero. On the Left Hand Side, note that , so the question now becomes when is greater or equal to zero.

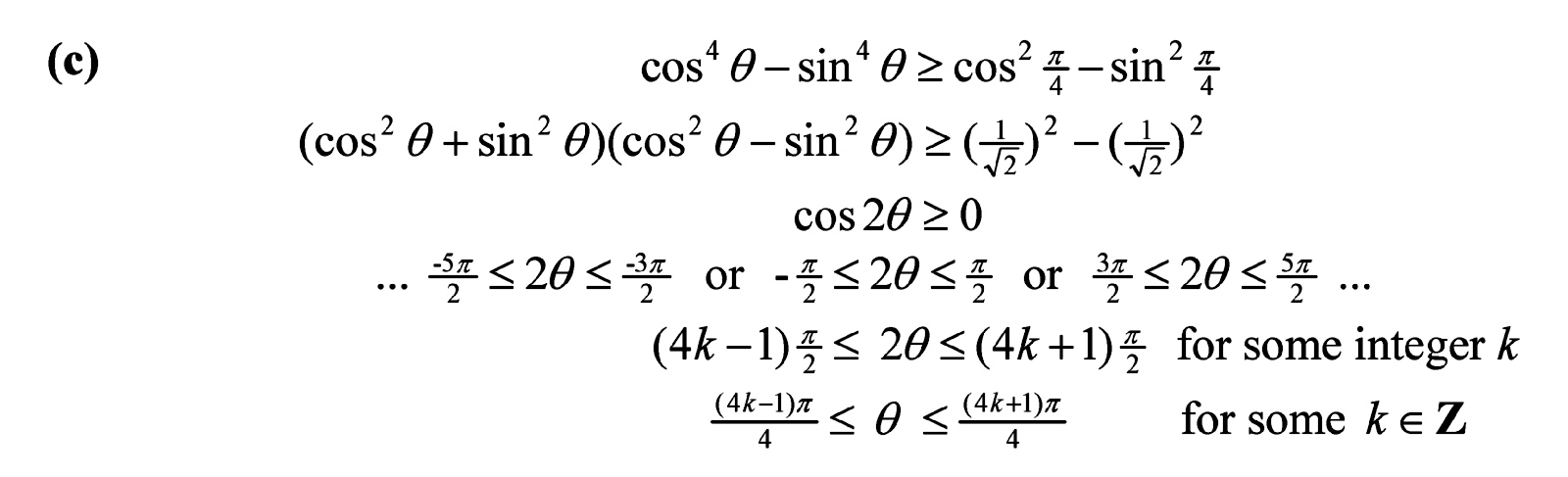
You may want to use the ASTC or "All Science Teachers are Crazy" mnemonic to help determine the quadrants where the cosine is positive or negative. For me, I prefer to consider the fact that the cosine is just the x-coordinate on the unit circle, so the cosine is positive on the right two quadrants (quadrants 1 and 4). We know that is non-negative from -90 degrees to 90 degrees, or from to radians. But we can go round and round the circle, clockwise and/or anti-clockwise indefinitely to get other solutions. This means we keep on adding or subtracting 360 degrees or radians. It turns out that there is a pattern. must be from to , where k is some integer. Dividing the inequality through by 2 gives our final answer.

In conclusion

When solving inequalities or equations, we should consider the possibility that the it may always be true, or never be true, or sometimes true.

I hope you have learned something solving these challenging inequalities.

Image Credits

The first picture was derived from Pixabay, while the rest are all my own work.

---

Announcements

You might also want to read my recent articles:
Integral of 1/sqrt(e^2x+1) (Trigo Approach)
Simplifying Roots
"Singapore Math": The Ribbons Problem from PSLE 2017

If you find my articles useful or interesting, please upvote and resteem them! Thanks !

Cheers!!!

@tradersharpe
-- promoting sharp minds